C. J. Shih This email address is being protected from spambots. You need JavaScript enabled to view it.1 and T. J. Tseng1 1Department of Mechanical Engineering Tamkang University Tamsui, Taipei, Taiwan, R.O.C.
Received:
March 12, 2001
Accepted:
March 29, 2001
Publication Date:
March 29, 2001
Download Citation:
||https://doi.org/10.6180/jase.2001.4.1.07
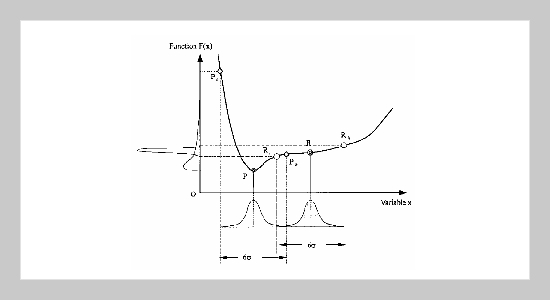
This paper presents an optimum design methodology for obtaining the highest robust performance using fuzzy multi-objective formulation strategy. The target performance and its variation, as functions of normally distributed variables with stochastic independence, are simultaneously minimized in this design process. A functional representation of the variability of the performance and the computational algorithm of the robust design process are presented in the paper. Two categories of design problems are examined: (1) the robust design with expected target of minimal variation. (2) The robust design with optimized target of minimal variation. The strength-based reliability behaves as the design objective that was merged in the formulation to extend the application of the proposed method. Three mechanical design examples further illustrate the presented integrated design methodology and successfully show its advantage.ABSTRACT
Keywords:
Robust Mechanical Design, Variability Optimization, Mean and Variance, Fuzzy Formulation, Engineering Design
REFERENCES