Free Asymmetric Transverse Vibration of Parabolically Varying Thickness Polar Orthotropic Annular Plate with Flexible Edge Conditions

R.P. Singh; S.K. Jain

doi:10.6180/jase.2004.7.1.07

Free Asymmetric Transverse Vibration of Parabolically Varying Thickness Polar Orthotropic Annular Plate with Flexible Edge Conditions

R. P. Singh ¹ and S. K. Jain²

¹Department of Applied Mechanics Birla Institute Technology Mesra, Ranchi−835215, India
²Department of Applied Mathematics Birla Institute Technology Mesra, Ranchi−835215, India

Received: June 14, 2003
Accepted: October 24, 2003
Publication Date: March 1, 2004

Download Citation: ||https://doi.org/10.6180/jase.2004.7.1.07

ABSTRACT

Free asymmetric transverse vibration of a polar orthotropic thin annular plate with parabolically varying thickness in radial direction have been computed on the basis of classical plate theory considering both the peripheries of the plate elastically restrained against translation and rotation. The fourth order linear differential equation with variable coefficients governing the motion in the present study has been solved using collocation interpolation technique taking Chebyshev points as the interpolating nodes. Frequency parameters of the plate considering some typical values of taper parameters, rigidity ratios and the radii ratios have been presented for the first three modes of vibration.

Keywords: Asymmetric Vibrations, Polar Orthotropic Annular Plate, Parabolically Varying Thickness, Chebyshev Points, Edges Elastically Restrained Against Translation and Rotation

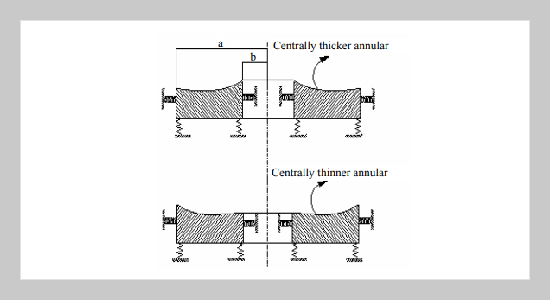
REFERENCES

[1] Lessia, W., NASA SP 160, Vibration of Plates (1969). [2] Ramaih, G. K. and Vijay Kumar, K. “Natural Frequencies of Polar Orthotropic Annular Plates,” Journal of Sound and Vibration, Vol. 26, p. 517 (1973).
[3] Woo, H. K., Kirmaser P. G. and Huang, C. L. “Vibration of Orthotropic Circular Plates with A Concentric Isotropic Core,” American Institute of Aeronautics and Astronautics Journal, Vol. 11, pp. 1421− 1422 (1973).
[4] Sinha Rao, K., Ganapathi, K. and Venkateswara Rao, G. “Vibration of Cylindrically Orthotropic Annular Plates,” Journal of Sound and Vibration, Vol. 36, pp. 433−434 (1974).
[5] Salzman, P. and Patel, S. A. ”Natural Frequencies of Orthotropic Annular Plates of Variable Thickness,” American institute of Aeronautics and Astronautics Journal, Vol. 6, pp. 1625−1626 (1968).
[6] Dyka, C. T. and Carney, J. F. “Vibrations of Annular Plates of Variable Thickness,” Journal of Engineering Mechanics, Vol. 105, pp. 361−370 (1979).
[7] Pardoen, G. “Vibration and Buckling Analysis of Axisymmetric Polar Orthotropic Annular Plates,” Computers and Structures, Vol. 4, pp. 951−960 (1973).
[8] Bell, R. and Kirkhope, J. “Vibration Analysis of Polar Orthotropic Discs Using the Transfer Matrix Method,” Journal and Sound and Vibration, Vol. 71, pp. 421− 428 (1980).
[9] Lal, R. and Gupta, U. S. “Axisymmetric Vibration of Polar Orthotropic Annular Plates of Variable Thickness,” Journal of Sound and Vibration, Vol. 83, pp. 229− 240 (1982).
[10] Gupta, U. S., Lal, R and Verma, C. P., “Buckling and Vibration of Polar Orthotropic Annular Plates on Elastic Foundation Subjected to Hydrostatic Peripheral Loading,” Journal of Sound and Vibration, Vol. 108, pp. 423−434 (1986).
[11] Kim, C. S. and Dickinson, S. M., “On the Lateral Vibration of Thin Annular and Circular Composite Plate Subject to Certain Complicating Effects,” Journal of Sound and Vibration, Vol. 130, pp. 363− 377 (1989).
[12] Kim, C. S. and Dickinson, S. M., “The Flectural Vibration of the Isotropic and Polar Orthotropic Annular and Circular Plates with Elastically Restrained Peripheries,” Journal of Sound and Vibration, Vol. 143, pp. 171−179 (1990).
[13] Gupta, U. S., Lal, R. and Jain, S. K., “Bulcking and Vibrations of Polar Orthotropic Circular Plates of Linearly Varying Thickness Resting on an Elastic Foundation,” Journal of Sound and Vibration, Vol. 147, pp. 423−434 (1991).
[14] Wang, X., Striz, A. G. and Bert, C. W., “Free Vibration Analysis of Annular Plates by the DQ Method,” Journal of Sound and Vibration, Vol. 164, pp. 173−175 (1993).
[15] Larrondo, H., Topalian, V., Avalos, D. R. and Laura, P. A. A., ”Free Vibration Analysis of Annular Plates by the DQ Method,” Journal of Sound and Vibration, Vol. 177, pp. 137−139 (1994).
[16] Wang, X., Yang, J. and Xian, J., “On Free Vibration Analysis of Circular Annular Plates with Non-uniform Thickness by the Differential Quadrature Method,” Journal of Sound and Vibration, Vol. 184, pp. 547−551 (1995).
[17] Avalos, D. R., Larrondo, H. K., Sanzogni, V. and Laura, P. A. A., “General, Approximate Solution of the Problem of Free Vibrations of Annular Plates of Nonuniform Thickness,” Institute of Applied Mechanics, Bahi Blanca, Argentina, (1995).
[18] Laura, P. A. A., Avalos, D. R., Larrondo, H. A. and Sonzogni, V., “Comments on Free Vibration Snalysis of Circular Annular Plates with Non-uniform Thickness by the Differential Quadrature Method,” Journal of Sound and Vibration, Vol. 195, pp. 338−339 (1996).
[19] Chen, D. Y., “Axisymmetric Vibration of Circular and Annular Plates with Arbitrarily Varying Thickness,” Journal of Sound and Vibration, Vol. 206, pp. 114− 121 (1997).
[20] Gutierrez, R. H., Laura, P. A. A., Felix, D. and Pistonesi, C., “Fundamental Frequency of Transverse Vibration of Circular Annular plates of Polar Orthotropy,” Journal of Sound and Vibration, Vol. 230, pp. 1191−1195 (2000).
[21] Lekhnitski, S. G. I, Anisotropic Plates, Gordon & Breach, NY, U.S.A. (1968).
[22] Conte and Boor, Elementary Numerical Analysis (An Algorithmic Approach), 3rd Edition, Mc Grow-Hill International, NY, U.S.A. (1985).