Chien-Hsun Lin This email address is being protected from spambots. You need JavaScript enabled to view it.¹ and Chan-Ping Pan¹

¹Department of Construction Engineering, National Taiwan University of Science and Technology, Taipei, Taiwan 106, R.O.C.

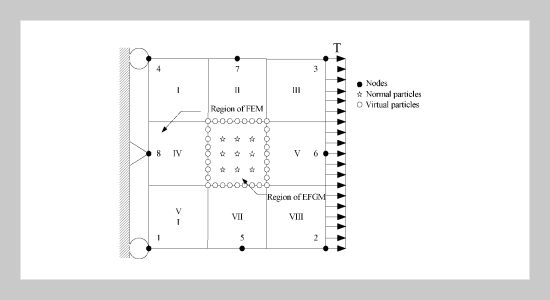
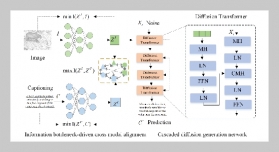
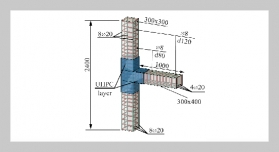
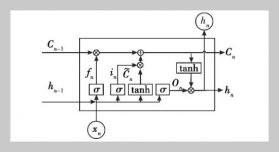
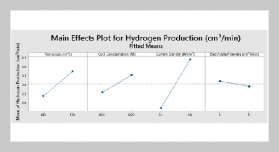
REFERENCES

1. [1] Belytschko, T., Lu, Y. Y. and Gu, L., “Element-Free Galerkin Methods,” International Journal for Numerical Methods in Engineering, Vol. 37, pp. 229
   256 (1994).
2. [2] Belytschko, T., Organ, D. and Krongauz, Y., “A Coupled Finite Element-Element Free Galerkin Method,” Computational Mechanics, Vol. 17, pp. 186
   195 (1995).
3. [3] Hegen, D., “Element-free Galerkin Methods in Combination with Finite Element Approaches,” Computer Methods in Applied Mechanics Engineering, Vol. 135, pp. 143
   166 (1996).
4. [4] Rao, B. N. and Rahman, S., “A Coupled MeshlessFinite Element Method for Fracture Analysis ofCracks,” International Journal of Pressure Vessels and Piping, Vol. 78, pp. 647
   657 (2001).
5. [5] Ho, S. L., Yang, S., Ni, G., Wong, H. C and Wang, Y., “Numerical Analysis of Thin Skin Depths of 3-D Eddy-Current Problems Using a Combination of Finite Element and Meshless Methods,” IEEE Transactions on Magnetics, Vol. 40, pp. 1354
   1357 (2004).
6. [6] Huerta, A. and Fernandez-Mendex, S., “Enrichment and Coupling of the Finite Element and Meshless Methods,” International Journal for Numerical Methods in Engineering, Vol. 48, pp. 1615
   1636 (2000).
7. [7] Lu, Y. Y., Belytschko, T. and Gu, L., “A New Implementation of the Element Free Galerkin Method,” Computer Methods in Applied Mechanics Engineering, Vol. 113, pp. 397
   414 (1994).
8. [8] Belytschko, T., Lu, Y. Y. and Gu, L., “Crack Propagation by Element-Free Galerkin Methods,” Engineering Fracture Mechanics, Vol. 51, pp. 295
   315 (1995).
9. [9] Krysl, P. and Belytschko, T., “Analysis of Thin Plates by the Element-Free Galerkin Methods,” Computational Mechanics, Vol. 17, pp. 26
   35 (1995).
10. [10] Fleming, M., Chu, Y. A., Moran, B. and Belytschko, T., “Enriched Element-Free Galerkin Methods for Crack Tip Fields,” International Journal for Numerical Methods in Engineering, Vol. 40, pp. 1483
    1504 (1997).
11. [11] Krongauz, Y. and Belytschko, T., “Enforcement of Essential Boundary Condition in Meshless Approximation Using Finite Elements,” Computer Methods in Applied Mechanics Engineering, Vol. 131, pp. 133
    145 (1996).
12. [12] Zhu, T. and Atluri, S. N., “A Modified Collocation Method and a Penalty Formulation for Enforcing the Essential Boundary Conditions in the Element Free Galerkin Method,” Computational Mechanics, Vol. 21, pp. 211
    222 (1998).
13. [13] Mukherjee, Y. X. and Mukherjee, S., “On Boundary Conditions in the Element-Free Galerkin Method,” Computational Mechanics, Vol. 19, pp. 264
    270 (1997).
14. [14] Gavete, L., Benito, J. J., Falcon, S. and Ruiz, A., “Implementation of Essential Boundary Conditions in a Meshless Method,” Communications in Numerical Methods in Engineering, Vol. 16, pp. 409
    421 (2000).
15. [15] Fernandez-Mendez, S. and Huerta, A., “Imposing Essential Boundary Conditions in Mesh-free Methods,” Computer Methods in Applied Mechanics Engineering, Vol. 193, pp. 1257
    1275 (2004).
16. [16] Lee, S. H. and Yoon, Y. C., “Numerical Prediction of Crack Propagation by an Enhanced Element-Free Galerkin Method,” Nuclear Engineering and Design, Vol. 227, pp. 257
    271 (2004).
17. [17] Gu, Y. T. and Liu, G. R., “A Coupled Element Eree Galerkin/Boundary Element Method for Stress Analysis of Two-Dimension Solids,” Computer Methods in Applied Mechanics Engineering, Vol. 190, pp. 4405
    4419 (2001).
18. [18] Rao, B. N. and Rahman, S., “An Enriched Meshless Method for Non-Linear Fracture Mechanics,” International Journal for Numerical Methods in Engineering, Vol. 59, pp. 197
    223 (2004).
19. [19] Cook, R. D., Malkus, D. S. and Plesha, M. E., Concepts and Applications of Finite Element Analysis, Third edition, John Wiley & Sons, New York (1989).
20. [20] Timoshenko, S. P. and Goodier, J. N., Theory of Elasticity, Third edition, McGraw-Hill, New York (1986).