Optimum Process Mean Setting for Product with Rework Process

Chung-Ho Chen; Michael B. C.  Khoo

doi:10.6180/jase.2010.13.4.03

Optimum Process Mean Setting for Product with Rework Process

Chung-Ho Chen ¹ and Michael B. C. Khoo²

¹Department of Management and Information Technology, Southern Taiwan University, Yungkang, Taiwan 710, R.O.C.
²School of Mathematical Sciences, Universiti Sains Malaysia, 11800 USM, Penang, Malaysia

Received: July 29, 2009
Accepted: April 22, 2010
Publication Date: December 1, 2010

Download Citation: ||https://doi.org/10.6180/jase.2010.13.4.03

ABSTRACT

The determination of the optimum process mean has been a major topic in statistical process control. It directly affects the process defective rate, production cost, scrap cost, and rework cost. In 2000, Lee et al. presented a filling problem for determining the optimum process mean and screening limits. They considered three grades of product, assumed a normal quality characteristic, and adopted the piecewise linear profit function for measuring the profit per item. However, they have not included the scrap cost and the perfect rework process in their model. In this paper, we further propose a modified Lee et al.’s model with rework process for determining the optimum process mean. Both perfect rework and imperfect rework processes for the product are considered in the model.

Keywords: Process Mean, Process Variance, Rework Process

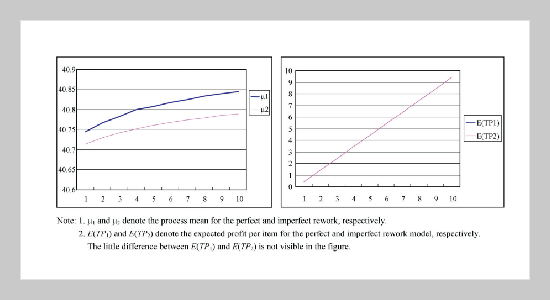
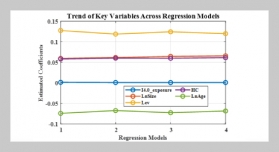
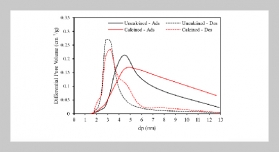
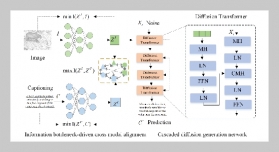
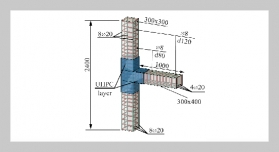
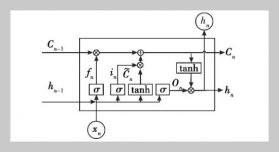
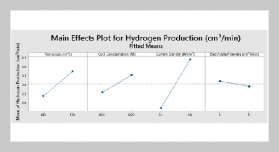
REFERENCES

[1] Li, M.-H. C., “Optimal setting of the process mean for asymmetrical quadratic quality loss function,” Proceedings of the Chinese Institute of Industrial Engineers Conference, pp. 415-419 (1997).
[2] Li, M.-H. C., “Optimal setting of the process mean for an asymmetrical truncated loss function,” Proceedings of the Chinese Institute of Industrial Engineers Conference, pp. 532-537 (1998).
[3] Li, M.-H. C., “Quality loss function based manufacturing process setting models for unbalanced tolerance design,” International Journal of Advanced Manufacturing Technology, Vol. 16, pp. 39-45 (2000).
[4] Li, M.-H. C., “Unbalanced tolerance design and manufacturing setting with asymmetrical linear loss function,” International Journal of Advanced Manufacturing Technology, Vol. 20, pp. 334-340 (2002).
[5] Li, M.-H. C., “Optimal process setting for unbalanced tolerance design with linear loss function,” Journal of the Chinese Institute of Industrial Engineers, Vol. 19,17-22 (2002).
[6] Li, M.-H. C. and Chirng, H.-S., “Optimal setting of the process mean for asymmetrical linear quality loss function,” 1999 Conference on Technology and Applications of Quality Management for Twenty-first Century, pp. 6-11 (1999)
[7] Li, M.-H.C. and Cherng, H.-S., “Unbalanced tolerance design with asymmetric truncated linear loss function,” The 14th Asia Quality Symposium, pp.162-165 (2000).
[8] Li, M.-H. C. and Chou, C.-Y., “Target selection for an indirectly measurable quality characteristic in unbalanced tolerance design,” International Journal of Advanced Manufacturing Technology, Vol. 17, pp. 516-522 (2001).
[9] Li, M.-H. C. and Wu, F.-W., “A general model of unbalanced tolerance design and manufacturing setting with asymmetric quadratic loss function,” Proceeding of Conference of the Chinese Society for Quality, pp. 403-409 (2001)
[10] Li, M.-H. C. and Wu, F.-W., “A general model of manufacturing setting with asymmetric linear loss function,” The 38th Annual Conference of Chinese Society for Quality, pp.1137-1143 (2002).
[11] Wu, C.C. and Tang, G.R., “Tolerance design for products with asymmetric quality losses,” International Journal of Production Research, Vol. 39, pp. 2529-2541 (1998).
[12] Maghsoodloo, S. and Li, M.-H. C., “Optimal asymmetrical tolerance design,” IIE Transactions, Vol. 32, pp. 1127-1137 (2000).
[13] Phillips, M. D. and Cho, B.-R., “A nonlinear model for determining the most economic process mean under a beta distribution,” International Journal of Reliability, Quality and Safety Engineering, Vol. 7, pp. 61-74 (2000).
[14] Springer, C. H., “A method of determining the most economic position of a process mean,” Industrial Quality Control, Vol. 8, pp. 36-39 (1951).
[15] Hunter, W. G., and Kartha, C. P., “Determining the most profitable target value for a production process,” Journal of Quality Technology, Vol. 9, pp. 176-181 (1977)
[16] Carlsson, O., “Determining the most profitable process level for a production process under different sales conditions,“ Journal of Quality Technology, Vol. 16, pp. 44-49 (1984).
[17] Carlsson, O., “Economic selection of a process level under acceptance sampling by variables,” Engineering Costs and Production Economics, Vol. 16, pp. 69-78 (1989)
[18] Bisgaard, S., Hunter, W. G., and Pallesen, L., “Economic selection of quality of manufactured product,” Technometrics, Vol. 26, pp. 9-18 (1984).
[19] Golhar, D. Y., “Determination of the best mean contents for a ‘Canning Problem’,” Journal of Quality Technology, Vol. 19, pp. 82-84 (1987).
[20] Golhar, D. Y., “Computation of the optimal process mean and the upper limit for a canning problem,” Journal of Quality Technology, Vol. 20, pp.193-195 (1988).
[21] Golhar, D. Y. and Pollock, S. M., “Determination of the optimal process mean and the upper limit of the canning problem,” Journal of Quality Technology, Vol. 20, pp. 188-192 (1988)
[22] Golhar, D. Y. and Pollock, S. M., “Cost savings due to variance reduction in a canning process,” IIE Transactions, Vol. 24, pp. 88-92 (1992).
[23] Rahim, M. A. and Banerjee, P. K., “Optimal production run for a process with random linear drift,” Omega, Vol. 16, pp. 347-351 (1988).
[24] Arcelus, F. J. and Rahim, M. A., “Optimal process levels for the joint control of variables and attributes,” European Journal of Operational Research, Vol. 45, 224-230 (1990)
[25] Boucher, T. O. and Jafari, M. A., “The optimum target value for single filling operations with quality sampling plans,” Journal of Quality Technology, Vol. 23, 44-47 (1991).
[26] Al-Sultan, K. S., “An algorithm for the determination of the optimum target values for two machines in series with quality sampling plans,” International Journal of Production Research, Vol. 32, pp. 37-45 (1994).
[27] Pulak, M. F. S. and Al-Sultan, K. S., “The optimum targeting for a single filling operation with rectifying inspection,” Omega, Vol. 24, pp. 727-733 (1996).
[28] Al-Sultan, K. S. and Al-Fawzan, M. A., “Variance reduction in a process with random linear drift,” International Journal of Production Research, Vol. 35, pp.1523-1533 (1997).
[29] Al-Sultan, K. S. and Pulak, M. F. S., “Process improvement by variance reduction for a single filling operation with rectifying inspection,” Production Planning & Control, Vol. 8, pp. 431-436 (1997).
[30] Lee, M. K. and Jang, J. S., “The optimum target values for a production process with three-class screening,” International Journal of Production Economics, Vol. 49, pp. 91-99 (1997).
[31] Misiorek, V. I. And Barrnett, N. S., “Mean selection for filling processes under weights and measures requirements,” Journal of Quality Technology, Vol. 32, 111-121 (2000).
[32] Lee, M. K. and Elsayed, E. A., “Process mean and screening limits for filling processes under two-stage screening procedure,” European Journal of Operational Research, Vol. pp. 138, 118-126 (2002).
[33]. Lee, M. K., Hong, S. H., Kwon, H. M., and Kim, S. B., “Optimum process mean and screening limits for a production process with three-class screening,” International Journal of Reliability, Quality and Safety Engineering, Vol. 7,179-190 (2000).
[34] Lee, M. K., Hong, S. H., and Elsayed, E. A., “The optimum target value under single and two-stage screenings,” Journal of Quality Technology, Vol. 33, pp.506-514 (2001).
[35] Duffuaa, S. O. and Siddiqi, A. W., “Integrated process targeting and product uniformity model for three-class screening,” International Journal of Reliability, Quality and Safety Engineering, Vol. 9, pp. 261-274 (2002).
[36] Taguchi, G., Introduction to Quality Engineering, Asian Productivity Organization, Tokyo, Japan (1986).