Finite Element Analysis of Fully Developed Unsteady MHD Convection Flow in a Vertical Rectangular Duct with Viscous Dissipation and Heat Source/Sink

Naikoti Kishan; Balla Chandra Shekar

doi:10.6180/jase.2015.18.2.06

Finite Element Analysis of Fully Developed Unsteady MHD Convection Flow in a Vertical Rectangular Duct with Viscous Dissipation and Heat Source/Sink

Naikoti Kishan This email address is being protected from spambots. You need JavaScript enabled to view it.¹ and Balla Chandra Shekar¹

¹Department of Mathematics, Osmania University, Hyderabad, Telangana State, India

Received: November 13, 2014
Accepted: April 29, 2015
Publication Date: June 1, 2015

Download Citation: ||https://doi.org/10.6180/jase.2015.18.2.06

ABSTRACT

The combined effect of viscous and Ohmic dissipations on unsteady, laminar magneto convection fully developed flow in a vertical rectangular duct considering the effects of heat source/ sink is investigated. Finite element method based on Galerkin weighted residual approach is used to solve two dimensional governing momentum and energy equations for unsteady, magneto convection flow in a vertical rectangular duct. The investigations are conducted for the effects of various flow parameters such as buoyancy parameter N, Hartmann number M, aspect ratio A, circuit parameter E and heat source/sink parameter λ. The results indicate that the flow pattern and the temperature field are significantly dependent on the above mentioned parameters. It is shown that buoyancy parameter, Hartmann number and aspect ratio parameter increase both the velocity and temperature for open circuit (E ≠ 0) but decrease for short circuit (E = 0).

Keywords: MHD, Fully Developed, Rectangular Duct, Heat Source/Sink, Finite Element Method, Electric Field

REFERENCES

[1] Aung, W. and Worku, G., “Theory of Fully Developed Combined Convection Including Flow Reversal,” ASME J Heat Transf., Vol. 108, pp. 485488 (1986). doi: 10. 1115/1.3246958
[2] Aung, W. and Worku, G., “Developing Flow and Flow Reversal in a Vertical Channel with Asymmetric Wall Temperature,” ASME J Heat Transf., Vol. 108, pp. 299304 (1986). doi: 10.1115/1.3246919
[3] Giuseppe, S. and Michele, C., “Fully Developed Mixed Magnetohydrodynamic Convection in a Vertical Square Duct,” Numer. Heat Transfer A., Vol. 53, pp. 907924 (2008). doi: 10.1080/00397910601149934
[4] Chang, C. C. and Lundgren, T. S., “Duct Flow in Magnetohydrodynamics,” Z. Angew. Math. Phys. (ZAMP), Vol. 12, pp. 100114 (1961). doi: 10.1007/BF01601011
[5] Hunt, J. C. R., “Magnetohydrodynamic Flow in a Rectangular Duct,” J. Fluid Mech., Vol. 21, No. 4, pp. 577590 (1965). doi: 10.1017/S0022112065000344
[6] Uflyand, Y. S., “Flow of Conducting Fluid in a Rectangular Channel in a Transverse Magnetic Field,” Sov. Phys. Tech. Phys., Vol. 5, p. 1194 (1961). doi: 10.1063/ 1.3624837
[7] Romig, M., “The Influence of Electric and Magnetic Field on Heat Transfer to Electrically Conducting Fluids,” Adv Heat Transf., Vol. 1, pp. 268352 (1964). doi: 10.1016/S0065-2717(08)70100-X
[8] Sutton, G. W. and Sherman, A., Engineering Magnetohydrodynamic, McGraw-Hill, New York (1965).
[9] Alpher, R. A., “Heat Transfer in Magnetohydrodynamic Flow between Parallel Plates,” Int J Heat Mass Transf., Vol. 3, pp. 108112 (1960). doi: 10.1016/0017- 9310(61)90073-4
[10] Nigam, S. D. and Singh, S. N., “Heat Transfer by Laminar Flow between Parallel Plates under the Action of Transverse Magnetic Field,” Q J Mech Appl Math., Vol. 13, pp. 8597 (1960). doi: 10.1093/qjmam/13.1.85
[11] Winter, H. H., “Viscous Dissipation in Shear Flows of Polymers,” Adv Heat Transf., Vol. 13, pp. 205267 (1977). doi: 10.1016/S0065-2717(08)70224-7
[12] Shadid, J. N. and Eckert, E. R. G., “Viscous Heating of a Cylinder with Finite Length by a High Viscosity Fluid in Steady Longitudinal Flow,” Int J Heat Mass Transf., Vol. 35, pp. 27392749 (1992). doi: 10.1016/ 0017-9310(92)90114-8
[13] Buhler, L., “Laminar Buoyant Magnetohydrodynamic Flow in a Rectangular Duct,” Phys Fluids., Vol. 10, pp. 223236 (1998). doi: 10.1063/1.869562
[14] Yakubenko, A. E., “Calculation of the Flow of an Electrically Conducting Liquid in a Duct of Rectangular Cross Section with Electrodes,” Fluid Dynamic, Vol. 5, pp. 748751 (1970). doi: 10.1007/BF01017291
[15] Prabal, T. and Mitesh, S., “Analysis of Laminar Mixed Convective Heat Transfer in Horizontal Triangular Ducts,” Numerical Heat Transfer, Part A., Vol. 54, pp. 11481168 (2008). doi: 10.1080/10407798508552113
[16] Sparrow, E. M. and Cess, R. D., “Temperature Dependent Heat Sources or Sinks in a Stagnation Point Flow,” Appl Sci Res A., Vol. 10, pp. 185197 (1961). doi: 0.1007/BF00411912
[17] Chamkha, A. J., “On Laminar Hydromagnetic Mixed Convection Flow in a Vertical Channel with Symmetric and Asymmetric Wall Heating Conditions,” Int Commun Heat Mass Transf., Vol. 45, pp. 25092525(2002). doi: 10.1016/S0017-9310(01)00342-8
[18] Jha, B. K. and Ajibade, A. O., “Free Convective Flow of Heat Generating/Absorbing Fluid between Vertical Porous Plates with Periodic Heat Input,” Int. Comm. Heat Mass Transfer., Vol. 36, p. 624 (2009). doi: 10. 1016/j.icheatmasstransfer.2009.03.003
[19] Kamel, M. H., “Unsteady MHD Convection through Porous Medium with Combined Heat and Mass Transfer with Heat Source/Sink,” Energy Conversion and Management, Vol. 42, p. 393 (2001). doi: 10.1016/ S0196-8904(00)00067-4
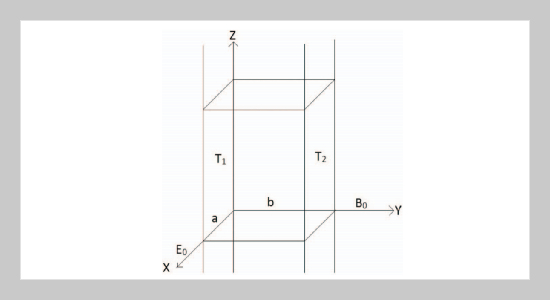
[20] Umavathi, J. C., Liu, I. C., Prathap Kumar, J. and Pop, I., “Fully Developed Magneto Convection Flow in a Vertical Rectangular Duct,” Heat Mass Transfer, Vol. 47, pp. 111 (2011). doi: 10.1007/s00231-010-0650-2
[21] Umavathi, J. C. and Liu, I. C., “Magnetoconvection in a Vertical Channel with Heat Source or Sink,” Meccanica, Vol. 48, pp. 22212232 (2013). doi: 10.1007/ s11012-013-9739-2
[22] Hughes, W. F. and Young, F. J., The Electro Magneto Hydrodynamics of Fluids, Wiley, New York (1996).