REFERENCES
- [1] Sabatier,P.C.,“TheoreticalConsiderations for Inverse Scattering,” Radio Science, Vol. 18, No. 1, pp. 629– 631 (1983). doi: 10.1029/RS018i001p00001
- [2] Storn, R. and Price, K., “Differential Evolution - a Simple and Efficient Adaptive Scheme for Global Optimization over Continuous Spaces,” Technical Report TR-95-012, International Computer Science Institute, Berkeley, Mar. (1995).
- [3] Kennedy,J.and Eberhart, R.C.,“Particle Swarm Optimization,”Proceedings of the IEEE International Conference on Neural Network, pp. 1942–1948 (1995). doi: 10.1109/ICNN.1995.488968
- [4] Rekanos, I. T., “Shape Reconstruction of a Perfectly Conducting Scatterer Using Differential Evolution and Particle Swarm Optimization,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 46, No. 7, pp. 1967–1974 (2008). doi: 10.1109/TGRS.2008.916635
- [5] Michalski, K. A., “Electromagnetic Imaging of Circular-cylindrical Conductors and Tunnels Using a Differential Evolution Algorithm,” Microwave and Optical Technology Letters, Vol. 27, No. 5, pp. 330–334 (2000). doi: 10.1002/1098-2760(20001205)27:5<330 ::AID-MOP13>3.0.CO;2-H
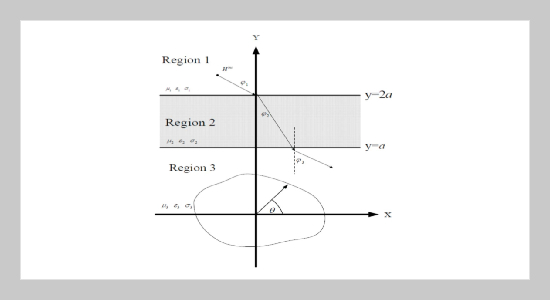
- [6] Lee, Y. H., Cheng, Y. T., Chiu, C. C. and Chang, S. P., “Microwave Imaging for Half-space Imperfect Conductors,” Nondestructive Testing and Evaluation, Vol. 30, No. 1, pp. 49�62 (2015). (EI) (SCI) doi: 10.1080/10589759.2014.992430
- [7] Chiang, J. S., Gu, W. S., Chiu, C. C. and Sun, C. H., “Estimation of the Two-dimensional Homogenous Dielectric Scatterer in a Slab Medium Using Particle Swarm Optimization and Asynchronous Particle Swarm Optimization,” Research in Nondestructive Evaluation, Vol. 26, No. 4, pp. 208–224 (2015). doi: 10.1080/ 09349847.2015.1024906
- [8] Yu, C. Y., Chiu, C. C., Chou, Y. K. and Shen, S. C., “Microwave Imaging in Frequency Domain for Through-wall Multiple Conductors,” Journal of Testing and Evaluation, Vol. 44, No. 4, pp. 1617�1623 (2016). (EI) (SCI) doi: 10.1520/JTE20140237
- [9] Ao, Y. Y. and Chi, H. Q., “Dynamic Differential Evolution for Constrained Real-parameter Optimization,” Journal of Advances in Information Technology, Vol. 1,No. 1,pp. 43–51(2010). doi:10.4304/jait.1.1.43-51
- [10] Cheng, Y. T., Chiu, C. C., Chang, S. P. and Hsu, J. C., “Comparison of Particle Swarm Optimization and Self-adaptive Dynamic Differential Evolution for the Imaging of a Periodic Conductor,” International Journal of Applied Electromagnetics and Mechanics, Vol. 46, No. 1, pp. 69–79 (2014).
- [11] Sun, C. H. and Chiu, C. C., “Inverse Scattering of Dielectric Cylindrical Target Using Dynamic Differential Evolution and Self-adaptive Dynamic Differential Evolution,” International Journal of RF and Microwave Computer-Aided Engineering,Vol.23, No. 5, pp. 579–585 (2013). doi: 10.1002/mmce.20692
- [12] Gennarelli, G., Vivone, G. and Braca, P., “Multiple Extended Target Tracking for Through-wall Radars,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 53, No. 12, pp. 6482–6494 (2015). doi: 10. 1109/TGRS.2015.2441957
- [13] Tivive, F., Bouzerdoum, A. and Amin, M., “A Subspace Projection Approach for Wall Clutter Mitigation in Through-the-wall Radar Imaging,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 53, No. 4, pp. 2108–2122 (2015). doi: 10.1109/TGRS.2014. 2355211
- [14] Dehmollaian, M. and Sarabandi, K., “Refocusing through Building Walls Using Synthetic Aperture Radar,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 46, No. 6, pp. 1589–1599 (2008).
- [15] Wang, Y. and Fathy, A. E., “Advanced System Level Simulation Platform for Three-dimensional UWB Through-wall Image,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 50, No. 5, pp. 1986–2000 (2012). doi: 10.1109/TGRS.2011.2170694
- [16] Zhene, W., Zhao, Z. and Nie, Z., “Application of TRM in the UWB through wall radar,” Prog. Electromagn., Res., Vol. 87, pp. 279–296 (2008). doi: 10.2528/ PIER08101202
- [17] Gennarelli, G. and Soldovieri, F., “Radar Imaging through Cinderblock Walls: Achievable Performance by a Model-corrected Linear Inverse Scattering Approach,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 52, No. 10, pp. 6738–6749 (2014). doi: 10.1109/TGRS.2014.2301851
- [18] Wan, Y., Yu, C. Y., Sun, C. H. and Chiu, C. C., “The Reconstruction of Time Domain Through-wall Imaging for a MetallicCylinder,” Imaging Science Journal, Vol. 63, No. 2, pp. 81–84 (2015). doi: 10.1179/ 1743131X14Y.0000000084
- [19] Lianlin, L., Zhang, W. and Li, F., “A Novel Autofocusing Approach for Real-time Through-wall Imaging under Unknown Wall Characteristics,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 48, No. 1, pp. 423–431 (2010). doi: 10.1109/TGRS.2009. 2024686
- [20] Kong, L. J., Cui, G. L., Yang, J. Y. and Yang, X. B., “Wall Parameters Estimation Method for Throughthe-wall Radar Imaging,” International Conference on Radar, pp. 297–301 (2008).
- [21] Li, C. L., Sun, C. H., Chiu, C. C. and Tuen, L. F., “Solving Inverse Scattering for a Partially Immersed Metallic Cylinder Using Steady-state Genetic Algorithm and Asynchronous Particle Swarm Optimization by TE Waves,” The Applied Computational ElectromagneticsSociety,Vol.28, No. 8,pp. 663–671 (2013).
- [22] Shen, J., Zhong, Y., Chen, X. and Ran, L., “Inverse Scattering Problems of Reconstructing Perfectly Electric Conductors with TE Illumination,” IEEE Transactions on Antennas and Propagation, Vol. 61, No. 9, pp. 4713–4721 (2013). doi: 10.1109/TAP.2013.2271891
- [23] Brest, J., Greiner, S., Boskovic, B., Mernik, M. and Zumer, V., “Self-adapting Control Parameters in Differential Evolution: Comparative Study on Numerical Benchmark Problems,” IEEE Transactions on Evolutionary Computation, Vol. 10, No. 6, pp. 646–657 (2006). doi: 10.1109/TEVC.2006.872133
- [24] Goudos, S. K., Siakavara, K., Samaras, T., Vafiadis, E. E. and Sahalos, J. N., “Self-adaptive Differential Evolution Applied to Real-valued Antenna and Microwave Design Problems,”IEEETransactions on Antennas and Propagation, Vol. 59, No. 4, pp. 1286–1298 (2011). doi: 10.1109/TAP.2011.2109678