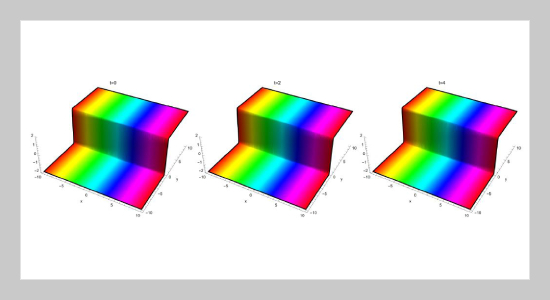
REFERENCES
- [1] A. D. Polyanin and V. G. Sorokin, (2021) “A method for constructing exact solutions of nonlinear delay PDEs" Journal of Mathematical Analysis and Applications 494(2): DOI: 10.1016/j.jmaa.2020.124619.
- [2] B. Ghanbari and A. Atangana, (2020) “Some new edge detecting techniques based on fractional derivatives with non-local and non-singular kernels" Advances in Difference Equations 2020(1): DOI: 10.1186/s13662-020-02890-9.
- [3] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2016) “Travelling wave solutions of nonlinear systems of PDEs by using the functional variable method" Boletim da Sociedade Paranaense de Matematica 34(2): 213–229. DOI: 10.5269/bspm.v34i2.25501.
- [4] S. Djilali and B. Ghanbari, (2021) “The influence of an infectious disease on a prey-predator model equipped with a fractional-order derivative" Advances in Difference Equations 2021(1): DOI: 10.1186/s13662-020-03177-9.
- [5] M. Eslami and H. Rezazadeh, (2016) “The first integral method for Wu–Zhang system with conformable time fractional derivative" Calcolo 53(3): 475–485. DOI: 10.1007/s10092-015-0158-8.
- [6] B. Ghanbari, (2020) “A fractional system of delay differential equation with nonsingular kernels in modeling hand-foot-mouth disease" Advances in Difference Equations 2020(1): DOI: 10.1186/s13662-020-02993-3.
- [7] B. Ghanbari, (2021) “Chaotic behaviors of the prevalence of an infectious disease in a prey and predator system using fractional derivatives" Mathematical Methods in the Applied Sciences 44(13): 9998–10013. DOI: 10.1002/mma.7386.
- [8] B. Ghanbari, (2020) “On approximate solutions for a fractional prey-predator model involving the Atangana–Baleanu derivative" Advances in Difference Equations 2020(1): DOI: 10.1186/s13662-020-03140-8.
- [9] A. Nabti and B. Ghanbari, (2021) “Global stability analysis of a fractional SVEIR epidemic model" Mathematical Methods in the Applied Sciences 44(11): 8577–8597. DOI: 10.1002/mma.7285.
- [10] M. S. M. Shehata, H. Rezazadeh, E. H. M. Zahran, E. Tala-Tebue, and A. Bekir, (2019) “New Optical Soliton Solutions of the Perturbed Fokas-Lenells Equation" Communications in Theoretical Physics 71(11): 1275–1280. DOI: 10.1088/0253-6102/71/11/1275.
- [11] H. Rezazadeh, (2018) “New solitons solutions of the complex Ginzburg-Landau equation with Kerr law nonlinearity" Optik 167: 218–227. DOI: 10.1016/j.ijleo.2018.04.026.
- [12] H. Rezazadeh, D. Kumar, T. A. Sulaiman, and H. Bulut, (2019) “New complex hyperbolic and trigonometric solutions for the generalized conformable fractional Gardner equation" Modern Physics Letters B 33(17): DOI: 10.1142/S0217984919501963.
- [13] B. Ghanbari and S. Djilali, (2020) “Mathematical and numerical analysis of a three-species predator-prey model with herd behavior and time fractional-order derivative" Mathematical Methods in the Applied Sciences 43(4): 1736–1752. DOI: 10.1002/mma.5999.
- [14] A. Alharbi and M. Almatrafi, (2020) “Numerical investigation of the dispersive long wave equation using an adaptive moving mesh method and its stability" Results in Physics 16: DOI: 10.1016/j.rinp.2019.102870.
- [15] B. Ghanbari, (2020) “On the modeling of the interaction between tumor growth and the immune system using some new fractional and fractional-fractal operators" Advances in Difference Equations 2020(1): DOI: 10.1186/s13662-020-03040-x.
- [16] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2015) “Exact solutions for the fractional differential equations by using the first integral method" Nonlinear Engineering 4(1): 15–22. DOI: 10.1515/nleng-2014-0018.
- [17] M. Inc, A. Yusuf, A. I. Aliyu, and D. Baleanu, (2017) “Optical soliton solutions for the higher-order dispersive cubic-quintic nonlinear Schrödinger equation" Superlattices and Microstructures 112: 164–179. DOI: 10.1016/j.spmi.2017.08.059.
- [18] S. T. R. Rizvi, K. Ali, and H. Hanif, (2019) “Optical solitons in dual core fibers under various nonlinearities" Modern Physics Letters B 33(17): DOI: 10.1142/S0217984919501896.
- [19] J. Hu and H. Zhang, (2001) “A new method for finding exact traveling wave solutions to nonlinear partial differential equations" Physics Letters, Section A: General, Atomic and Solid State Physics 286(2-3): 175–179. DOI: 10.1016/S0375-9601(01)00291-2.
- [20] L. Wazzan, (2009) “A modified tanh–coth method for solving the KdV and the KdV–Burgers’ equations" Communications in Nonlinear Science and Numerical Simulation 14(2): 443–450.
- [21] B. Ghanbari and D. Baleanu, (2019) “A novel technique to construct exact solutions for nonlinear partial differential equations" European Physical Journal Plus 134(10): DOI: 10.1140/epjp/i2019-13037-9.
- [22] N. Sajid and G. Akram, (2020) “Novel solutions of Biswas-Arshed equation by newly ϕ6-model expansion method" Optik 211: DOI: 10.1016/j.ijleo.2020.164564.
- [23] B. Ghanbari, S. Kumar, M. Niwas, and D. Baleanu, (2021) “The Lie symmetry analysis and exact Jacobi elliptic solutions for the Kawahara–KdV type equations" Results in Physics 23: DOI: 10.1016/j.rinp.2021.104006.
- [24] H. Kim and R. Sakthivel, (2012) “New exact traveling wave solutions of some nonlinear higher-dimensional physical models" Reports on Mathematical Physics 70(1): 39–50.
- [25] Y. Li, D. Lu, M. Arshad, and X. Xu, (2021) “New exact traveling wave solutions of the unstable nonlinear Schrödinger equations and their applications" Optik 226: DOI: 10.1016/j.ijleo.2020.165386.
- [26] V. S. Erturk and P. Kumar, (2020) “Solution of a COVID-19 model via new generalized Caputo-type fractional derivatives" Chaos, Solitons and Fractals 139: DOI: 10.1016/j.chaos.2020.110280.
- [27] B. Ghanbari, M. Inc, and L. Rada, (2019) “Solitary wave solutions to the tzitzéica type equations obtained by a new efficient approach" Journal of Applied Analysis and Computation 9(2): 568–589. DOI: 10.11948/2156-907X.20180103.
- [28] R. H. Goodman, P. J. Holmes, and M. I. Weinstein, (2004) “Strong NLS soliton-defect interactions" Physica D: Nonlinear Phenomena 192(3-4): 215–248. DOI: 10.1016/j.physd.2004.01.021.
- [29] B. Ghanbari, (2021) “Abundant exact solutions to a generalized nonlinear Schrödinger equation with local fractional derivative" Mathematical Methods in the Applied Sciences 44(11): 8759–8774. DOI: 10.1002/mma.7302.
- [30] B. Ghanbari, (2022) “On the nondifferentiable exact solutions to Schamel’s equation with local fractional derivative on Cantor sets" Numerical Methods for Partial Differential Equations 38(5): 1255–1270. DOI: 10.1002/num.22740.
- [31] I. Herron, C. McCalla, and R. Mickens, (2020) “Traveling wave solutions of Burgers’ equation with time delay" Applied Mathematics Letters 107: DOI: 10.1016/j.aml.2020.106496.
- [32] B. Ghanbari, (2021) “On novel nondifferentiable exact solutions to local fractional Gardner’s equation using an effective technique" Mathematical Methods in the Applied Sciences 44(6): 4673–4685. DOI: 10.1002/mma.7060.
- [33] K. Munusamy, C. Ravichandran, K. S. Nisar, and B. Ghanbari, (2020) “Existence of solutions for some functional integrodifferential equations with nonlocal conditions" Mathematical Methods in the Applied Sciences 43(17): 10319–10331. DOI: 10.1002/mma.6698.
- [34] B. Ghanbari, K. S. Nisar, and M. Aldhaifallah, (2020) “Abundant solitary wave solutions to an extended nonlinear Schrödinger’s equation with conformable derivative using an efficient integration method" Advances in Difference Equations 2020(1): DOI: 10.1186/s13662-020-02787-7.
- [35] M. Goyal, H. Baskonus, and A. Prakash, (2020) “Regarding new positive, bounded and convergent numerical solution of nonlinear time fractional HIV/AIDS transmission model" Chaos, Solitons & Fractals 2020(139:110096):
- [36] B. Ghanbari, A. Yusuf, M. Inc, and D. Baleanu, (2019) “The new exact solitary wave solutions and stability analysis for the (2 + 1 ) -dimensional Zakharov–Kuznetsov equation" Advances in Difference Equations 2019(1): DOI: 10.1186/s13662-019-1964-0.
- [37] N. A. Kudryashov, (2020) “Traveling wave solutions of the generalized Gerdjikov–Ivanov equation" Optik 219: DOI: 10.1016/j.ijleo.2020.165193.
- [38] K. Ayub, M. Y. Khan, and Q. Mahmood-Ul-Hassan, (2017) “Solitary and periodic wave solutions of Calogero–Bogoyavlenskii–Schiff equation via exp-function methods" Computers and Mathematics with Applications 74(12): 3231–3241. DOI: 10.1016/j.camwa.2017.08.021.
- [39] M. Bruzón, M. Gandarias, C. Muriel, C. Ramírez, S. Saez, and F. Romero, (2003) “The Calogero-Bogoyavlenskii-Schiff equation in 2+1 dimensions" Theoretical and Mathematical Physics 137(1): 1367–1377. DOI: 10.1023/A:1026040319977.
- [40] Y.-Z. Peng, (2006) “New types of localized coherent structures in the Bogoyavlenskii-Schiff equation" International Journal of Theoretical Physics 45(9): 1779–1783. DOI: 10.1007/s10773-006-9139-7.
- [41] B. Li, Y. Chen, H. Xuan, and H. Zhang, (2003) “Symbolic computation and construction of soliton-like solutions for a breaking soliton equation" Chaos, Solitons and Fractals 17(5): 885–893. DOI: 10.1016/S0960-0779(02)00570-2.
- [42] S.-T. Chen and W.-X. Ma, (2018) “Lump solutions of a generalized Calogero–Bogoyavlenskii–Schiff equation" Computers & Mathematics with Applications 76(7): 1680–1685.
- [43] M. Bruzón, M. Gandarias, C. Muriel, C. Ramírez, S. Saez, and F. Romero, (2003) “The Calogero-Bogoyavlenskii-Schiff equation in 2+1 dimensions" Theoretical and Mathematical Physics 137(1): 1367–1377. DOI: 10.1023/A:1026040319977.
- [44] Z. Hammouch, T. Mekkaoui, and P. Agarwal, (2018) “Optical solitons for the Calogero-Bogoyavlenskii-Schiff equation in (2+1) dimensions with time-fractional conformable derivative" The European Physical Journal Plus 133(7): 1–6.
- [45] B. Ghanbari and M. Inc, (2018) “A new generalized exponential rational function method to find exact special solutions for the resonance nonlinear Schrödinger equation" European Physical Journal Plus 133(4): DOI: 10.1140/epjp/i2018-11984-1.
- [46] B. Ghanbari and C.-K. Kuo, (2021) “Abundant wave solutions to two novel KP-like equations using an effective integration method" Physica Scripta 96(4): DOI: 10.1088/1402-4896/abde5a.
- [47] W. Gao, B. Ghanbari, H. Günerhan, and H. M. Baskonus, (2020) “Some mixed trigonometric complex soliton solutions to the perturbed nonlinear Schrödinger equation" Modern Physics Letters B 34(3): DOI: 10.1142/S0217984920500347.
- [48] B. Ghanbari and J. Gómez-Aguilar, (2019) “Optical soliton solutions for the nonlinear Radhakrishnan-Kundu-Lakshmanan equation" Modern Physics Letters B 33(32): DOI: 10.1142/S0217984919504025.
- [49] B. Ghanbari and J. Gómez-Aguilar, (2019) “New exact optical soliton solutions for nonlinear Schrödinger equation with second-order spatio-temporal dispersion involving M-derivative" Modern Physics Letters B 33(20):1950235.
- [50] Z. Pinar and T. Özi¸s, (2015) “Observations on the class of "Balancing Principle" for nonlinear PDEs that can be treated by the auxiliary equation method" Nonlinear Analysis: Real World Applications 23: 9–16. DOI: 10.1016/j.nonrwa.2014.11.001.
- [51] M. M. Khater, M. S. Mohamed, and R. A. Attia, (2021) “On semi analytical and numerical simulations for a mathematical biological model; the time-fractional nonlinear Kolmogorov–Petrovskii–Piskunov (KPP) equation" Chaos, Solitons & Fractals 144: 110676.
- [52] W. Li, L. Akinyemi, D. Lu, and M. M. A. Khater, (2021) “Abundant traveling wave and numerical solutions of weakly dispersive long waves model" Symmetry 13(6): DOI: 10.3390/sym13061085.
- [53] S. Sahoo and S. Saha Ray, (2020) “Invariant analysis with conservation law of time fractional coupled Ablowitz–Kaup–Newell–Segur equations in water waves" Waves in Random and Complex Media 30(3): 530–543. DOI: 10.1080/17455030.2018.1540899.
- [54] A. Tripathy and S. Sahoo, (2021) “A novel analytical method for solving (2+ 1)-dimensional extended Calogero-Bogoyavlenskii-Schiff equation in plasma physics" Journal of Ocean Engineering and Science 6(4): 405–409.
- [55] Z. P. Izgi, F. N. Saglam, S. Sahoo, H. Rezazadeh, and L. Akinyemi, (2022) “A partial offloading algorithm based on intelligent sensing" International Journal of Modern Physics B 36(17): DOI: 10.1142/S0217979222500977.
- [56] S. Sahoo and A. Tripathy, (2022) “New exact solitary solutions of the (2+1)-dimensional Heisenberg ferromagnetic spin chain equation" European Physical Journal Plus 137(3): DOI: 10.1140/epjp/s13360-022-02609-7.
- [57] S. Sahoo and S. Saha Ray, (2019) “On the conservation laws and invariant analysis for time-fractional coupled Fitzhugh-Nagumo equations using the Lie symmetry analysis" European Physical Journal Plus 134(2): DOI: 10.1140/epjp/i2019-12440-6.
- [58] S. Sahoo and S. Saha Ray, (2019) “A novel approach for stochastic solutions of wick-type stochastic time-fractional Benjamin–Bona–Mahony equation for modeling long surface gravity waves of small amplitude" Stochastic Analysis and Applications 37(3): 377–387.
- [59] S. Sahoo, S. Saha Ray, and S. Das, (2017) “An efficient and novel technique for solving continuously variable fractional order mass-spring-damping system" Engineering Computations (Swansea, Wales) 34(8): 2815–2835. DOI: 10.1108/EC-04-2016-0145.
- [60] Y. Chu, M. M. A. Khater, and Y. Hamed, (2021) “Diverse novel analytical and semi-analytical wave solutions of the generalized (2+1)-dimensional shallow water waves model" AIP Advances 11(1): DOI: 10.1063/5.0036261.
- [61] M. M. Khater, A. E.-S. Ahmed, and M. El-Shorbagy, (2021) “Abundant stable computational solutions of Atangana–Baleanu fractional nonlinear HIV-1 infection of CD4+ T-cells of immunodeficiency syndrome" Results in Physics 22: DOI: 10.1016/j.rinp.2021.103890.
- [62] M. M. Khater, A. E.-S. Ahmed, S. Alfalqi, J. Alzaidi, S. Elbendary, and A. M. Alabdali, (2021) “Computational and approximate solutions of complex nonlinear Fokas–Lenells equation arising in optical fiber" Results in Physics 25: DOI: 10.1016/j.rinp.2021.104322.
- [63] M. M. A. Khater, K. S. Nisar, and M. S. Mohamed, (2021) “Numerical investigation for the fractional nonlinear space-time telegraph equation via the trigonometric Quintic B-spline scheme" Mathematical Methods in the Applied Sciences 44(6): 4598–4606. DOI: 10.1002/mma.7052.
- [64] M. M. A. Khater and B. Ghanbari, (2021) “On the solitary wave solutions and physical characterization of gas diffusion in a homogeneous medium via some efficient techniques" European Physical Journal Plus 136(4): DOI: 10.1140/epjp/s13360-021-01457-1.
- [65] M. M. A. Khater, M. S. Mohamed, and S. Elagan, (2021) “Diverse accurate computational solutions of the nonlinear Klein–Fock–Gordon equation" Results in Physics 23: DOI: 10.1016/j.rinp.2021.104003.
- [66] M. M. A. Khater, A. Bekir, D. Lu, and R. A. M. Attia, (2021) “Analytical and semi-analytical solutions for timefractional Cahn–Allen equation" Mathematical Methods in the Applied Sciences 44(3): 2682–2691. DOI:10.1002/mma.6951.
- [67] M. M. A. Khater and A. E.-S. Ahmed, (2021) “Strong langmuir turbulence dynamics through the trigonometric quintic and exponential b-spline schemes" AIMS Mathematics 6(6): 5896–5908. DOI: 10.3934/math.2021349.