Approximate Solutions Of Two-point Boundary Value Problems By The Weak-form Integral Equation Method

Meixiang Wang

doi:10.6180/jase.202310_26(10).0014

Approximate Solutions Of Two-point Boundary Value Problems By The Weak-form Integral Equation Method

Mathematics / Statistics

Meixiang Wang This email address is being protected from spambots. You need JavaScript enabled to view it.¹

¹Basic Teaching Department, Zheng zhou Tourism College, Zheng zhou 450009, Henan, China

Received: April 26, 2022
Accepted: June 29, 2022
Publication Date: February 21, 2023

Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.

Download Citation: ||https://doi.org/10.6180/jase.202310_26(10).0014

ABSTRACT

In this article, we use an effective computational method for solving the two-point boundary value problems (BVPs). In order to use the weak-form integral equation method, we firstly introduce the suitable adjoint test functions. Then, we derive weak form of the given BVP, by utilizing suitable trial functions. Finally, we impose a combination of exponential functions as the basis of solution space. Different types of boundary conditions (of Dirichlet and robin types) are investigated and stability of the method are numerically considered.

Keywords: Two-point boundary value problem; Weak-form integral equation method; Adjoint test functions; Fractional order exponential trial functions

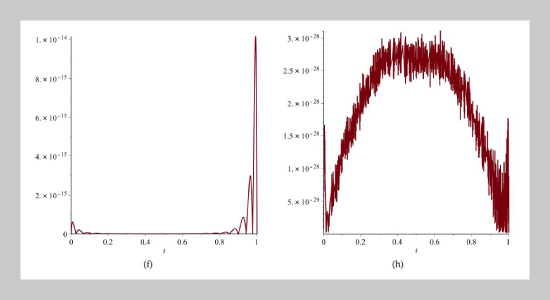
REFERENCES

[1] A. Akgül, M. Hashemi, M. Inc, and S. Raheem, (2017) “Constructing two powerful methods to solve the Thomas–Fermi equation" Nonlinear Dynamics 87(2): 1435–1444. DOI: 10.1007/s11071-016-3125-2.
[2] A. Akgül, M. Inc, and M. S. Hashemi, (2017) “Group preserving scheme and reproducing kernel method for the Poisson–Boltzmann equation for semiconductor devices" Nonlinear Dynamics 88(4): 2817–2829. DOI: 10.1007/s11071-017-3414-4.
[3] T. R. Mahapatra and A. Gupta, (2002) “Heat transfer in stagnation-point flow towards a stretching sheet" Heat and Mass Transfer/Waerme- und Stoffuebertragung 38(6): 517–521. DOI: 10.1007/s002310100215.
[4] M. S. Hashemi, H. Rezazadeh, H. Almusawa, and H. Ahmad, (2021) “A lie group integrator to solve the hydromagnetic stagnation point flow of a second grade fluid over a stretching sheet" AIMS Mathematics 6(12):13392–13406. DOI: 10.3934/math.2021775.
[5] M. Hashemi, (2017) “A novel simple algorithm for solving the magneto-hemodynamic flow in a semi-porous channel" European Journal of Mechanics, B/Fluids 65: 359–367. DOI: 10.1016/j.euromechflu.2017.05.008.
[6] M. Heydari, Z. Avazzadeh, and N. Hosseinzadeh, (2022) “Haar Wavelet Method for Solving High-Order Differential Equations with Multi-Point Boundary Conditions" Journal of Applied and Computational Mechanics 8(2): 528–544. DOI: 10.22055/jacm.2020.31860.1935.
[7] Z. Nikooeinejad, M. Heydari, and G. Loghmani, (2021) “Numerical solution of two-point BVPs in infinite horizon optimal control theory: a combined quasilinearization method with exponential Bernstein functions" International Journal of Computer Mathematics 98(11): 2156–2174. DOI: 10.1080/00207160.2021.1876850.
[8] Z. Nikooeinejad, M. Heydari, and G. Loghmani, (2022) “A numerical iterative method for solving two point BVPs in infinite-horizon nonzero-sum differential games: Economic applications" Mathematics and Computers in Simulation 200: 404–427. DOI: 10.1016/j.matcom.2022.04.022.
[9] M. Tafakkori–Bafghi, G. Loghmani, and M. Heydari, (2022) “Numerical solution of two-point nonlinear boundary value problems via Legendre–Picard iteration method" Mathematics and Computers in Simulation 199: 133–159. DOI: 10.1016/j.matcom.2022.03.022.
[10] M. Heydari and G. Loghmani, (2010) “Approximate solution to boundary value problems by the modified vim" Iranian Journal of Science and Technology, TransactionA: Science 34(2): 161–167.
[11] G. Adomian, (1988) “A review of the decomposition method in applied mathematics" Journal of Mathematical Analysis and Applications 135(2): 501–544. DOI:10.1016/0022-247X(88)90170-9.
[12] H. Yaghoobi and M. Torabi, (2011) “The application of differential transformation method to nonlinear equations arising in heat transfer" International Communications in Heat and Mass Transfer 38(6): 815–820. DOI:10.1016/j.icheatmasstransfer.2011.03.025.
[13] A. G. Deacon and S. Osher, (1979) “A finite element method for a boundary value problem of mixed type" SIAM Journal on Numerical Analysis 16(5): 756–778.
[14] S. Abbasbandy, E. Magyari, and E. Shivanian, (2009) “The homotopy analysis method for multiple solutions of nonlinear boundary value problems" Communications in Nonlinear Science and Numerical Simulation 14(9-10): 3530–3536. DOI: 10.1016/j.cnsns.2009.02.008.
[15] M. Dehghan, J. Manafian, and A. Saadatmandi, (2010) “The solution of the linear fractional partial differential equations using the homotopy analysis method" Zeitschrift fur Naturforschung - Section A Journal of Physical Sciences 65(11): 935–949. DOI: 10.1515/zna-2010-1106.
[16] M. Dehghan, J. Manafian, and A. Saadatmandi, (2010) “Solving nonlinear fractional partial differential equations using the homotopy analysis method" Numerical Methods for Partial Differential Equations 26(2): 448–479. DOI: 10.1002/num.20460.
[17] J. Manafian and C. Teymuri sindi, (2018) “An optimal homotopy asymptotic method applied to the nonlinear thin film flow problems" International Journal of Numerical Methods for Heat and Fluid Flow 28(12): 2816–2841. DOI: 10.1108/HFF-08-2017-0300.
[18] M. Hashemi and S. Abbasbandy, (2017) “A Geometric Approach for Solving Troesch’s Problem" Bulletin of the Malaysian Mathematical Sciences Society 40(1): 97–116. DOI: 10.1007/s40840-015-0260-8.
[19] M. Hashemi and A. Akgül, (2021) “On the MHD boundary layer flow with diffusion and chemical reaction over a porous flat plate with suction/blowing: two reliable methods" Engineering with Computers 37(2):1147–1158. DOI: 10.1007/s00366-019-00876-0.
[20] M. Hashemi, (2021) “Numerical study of the one-dimensional coupled nonlinear sine-Gordon equations by a novel geometric meshless method" Engineering with Computers 37(4): 3397–3407. DOI: 10.1007/s00366-020-01001-2.
[21] J. Manafian and R. Farshbaf Zinati, (2020) “Application of tan(Φ(ξ)/2) tan(Φ(ξ)/2)-expansion method to solve some nonlinear fractional physical model" Proceedings of the National Academy of Sciences India Section A - Physical Sciences 90(1): 67–86. DOI:10.1007/s40010-018-0550-2.
[22] D. Liu, X. Ju, O. A. Ilhan, J. Manafian, and H. F. Ismael, (2021) “Multi-Waves, Breathers, Periodic and Cross-Kink Solutions to the (2+1)-Dimensional Variable-Coefficient Caudrey-Dodd-Gibbon-Kotera-Sawada Equation" Journal of Ocean University of China 20(1): 35–44. DOI: 10.1007/s11802-021-4414-z.
[23] O. A. Ilhan, J. Manafian, A. Alizadeh, and S. A. Mohammed, (2020) “M lump and interaction between M lump and N stripe for the third-order evolution equation arising in the shallow water" Advances in Difference Equations 2020(1): DOI: 10.1186/s13662-020-02669-y.
[24] Y. Qian, J. Manafian, S. Y. Mohyaldeen, L. S. Esmail, S. A. Gorovoy, and G. Singh, (2021) “Multiple-order line rogue wave, lump and its interaction, periodic, and cross-kink solutions for the generalized CHKP equation" Propulsion and Power Research 10(3): 277–293. DOI:10.1016/j.jppr.2021.09.002.
[25] M. Eslami and H. Rezazadeh, (2016) “The first integral method for Wu–Zhang system with conformable time fractional derivative" Calcolo 53(3): 475–485. DOI: 10.1007/s10092-015-0158-8.
[26] H. Rezazadeh, D. Kumar, T. A. Sulaiman, and H. Bulut, (2019) “New complex hyperbolic and trigonometric solutions for the generalized conformable fractional Gardner equation" Modern Physics Letters B 33(17): DOI: 10.1142/S0217984919501963.
[27] M. S. M. Shehata, H. Rezazadeh, E. H. M. Zahran, E. Tala-Tebue, and A. Bekir, (2019) “New Optical Soliton Solutions of the Perturbed Fokas-Lenells Equation" Communications in Theoretical Physics 71(11): 1275–1280. DOI: 10.1088/0253-6102/71/11/1275.
[28] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2016) “Travelling wave solutions of nonlinear systems of PDEs by using the functional variable method" Boletim da Sociedade Paranaense de Matematica 34(2): 213–229. DOI: 10.5269/bspm.v34i2.25501.
[29] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2015) “Exact solutions for the fractional differential equations by using the first integral method" Nonlinear Engineering 4(1): 15–22. DOI: 10.1515/nleng-2014-0018.
[30] M. Dehghan and M. Abbaszadeh, (2016) “Analysis of the element free Galerkin (EFG) method for solving fractional cable equation with Dirichlet boundary condition" Applied Numerical Mathematics 109: 208–234. DOI: 10.1016/j.apnum.2016.07.002.
[31] M. Dehghan and R. Salehi, (2014) “A meshless local Petrov-Galerkin method for the time-dependent Maxwell equations" Journal of Computational and Applied Mathematics 268: 93–110. DOI: 10.1016/j.cam.2014.02.013.
[32] E. Shivanian, (2016) “On the convergence analysis, stability, and implementation of meshless local radial point interpolation on a class of three-dimensional wave equations" International Journal for Numerical Methods in Engineering 105(2): 83–110. DOI: 10.1002/nme.4960.
[33] L. Zhang, D. Huang, and K. Liew, (2015) “An elementfree IMLS-Ritz method for numerical solution of three-dimensional wave equations" Computer Methods in Applied Mechanics and Engineering 297: 116–139. DOI: 10.1016/j.cma.2015.08.018.
[34] L. Zhang and K. Liew, (2014) “An element-free based solution for nonlinear Schrödinger equations using the ICVMLS-Ritz method" Applied Mathematics and Computation 249: 333–345. DOI: 10.1016/j.amc.2014.10.033.
[35] C.-S. Liu and C.-W. Chang, (2018) “Solving nonlinear singularly perturbed problems by fractional order exponential trial functions" Applied Mathematics Letters 83: 219–226. DOI: 10.1016/j.aml.2018.04.008.
[36] C.-S. Liu, (2018) “Solving singularly perturbed problems by a weak-form integral equation with exponential trial functions" Applied Mathematics and Computation 329: 154–174. DOI: 10.1016/j.amc.2018.02.002.
[37] M. Jain, S. Sharma, and R. Mohanty, (2016) “High accuracy variable mesh method for nonlinear two-point boundary value problems in divergence form" Applied Mathematics and Computation 273: 885–896. DOI: 10.1016/j.amc.2015.10.030.
[38] R. Mohanty, (2005) “A family of variable mesh methods for the estimates of (du/dr) and solution of non-linear two point boundary value problems with singularity" Journal of Computational and Applied Mathematics 182(1): 173–187. DOI: 10.1016/j.cam.2004.11.045.
[39] R. Mohanty, D. Evans, and N. Khosla, (2005) “An O (hk3) non-uniform mesh cubic spline TAGE method for non-linear singular two-point boundary value problems" International Journal of Computer Mathematics 82(9): 1125–1139. DOI: 10.1080/00207160500112977.
[40] R. Mohanty and N. Khosla, (2006) “Application of TAGE iterative algorithms to an efficient third order arithmetic average variable mesh discretization for two-point non-linear boundary value problems" Applied Mathematics and Computation 172(1): 148–162. DOI: 10.1016/j.amc.2005.01.134.
[41] J. Ahlberg and T. Ito, (1975) “A collocation method for two point boundary value problems" Mathematics of Computation 29(131): 761–776. DOI: 10.1090/S0025-5718-1975-0375785-7.
[42] K. Raslan, M. Ramadan, and M. Shaalan, (2018) “THEORETICAL AND NUMERICAL STUDIES OF TWO POINT BOUNDARY VALUE PROBLEMS USING TRIGONOMETRIC AND EXPONENTIAL CUBIC B-SPLINES" Journal of the Egyptian Mathematical Society 26(2): 259–268.