Che Wun Chiou This email address is being protected from spambots. You need JavaScript enabled to view it.¹ and Liuh Chii Lin¹

¹Department of Electronic Engineering Ching Yun University Chungli, Taiwan 320, R.O.C.

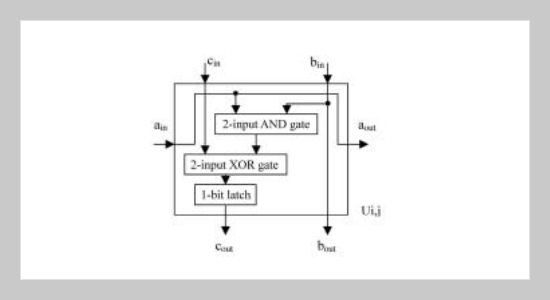
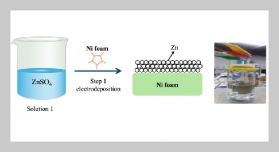
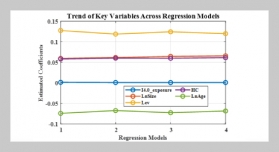
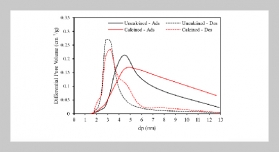
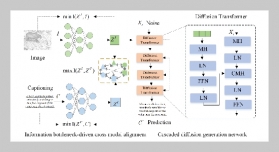
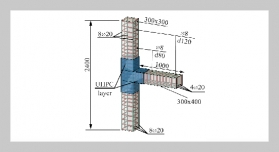
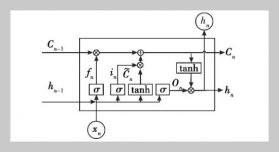
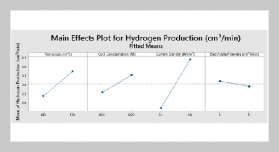
REFERENCES

1.  [1] MacWilliams, F. J. and Sloane, N. J. A., The Theory of Error-Correcting Codes, Amsterdam: North-Holland (1977).
2. [2] Peterson, W. W. and Weldon, Jr., E. J., ErrorCorrecting Codes, 2nd ed. Cambridge, MA: MIT Press (1972).
3. [3] Berlekamp, E. R., Algebraic Coding Theory, New York: McGraw-Hill (1968).
4. [4] Lidl, R. and Niederreiter, H., Introduction to Finite Fields and Their Applications, New York: Cambridge Univ. Press (1994).
5. [5] Application of Finite Fields, Menezes, A. J., ed. Boston: Kluwer Academic (1993).
6. [6] Yeh, C. S., Reed, S. and Truong, T. K., “Systolic Multipliers for Finite Fields GF(2m),” IEEE Trans. Computers, Vol. 33, pp. 357
   360 (1984).
7. [7] Massey, J. L. and Omura, J. K., “Computational Method and Apparatus for Finite Field Arithmetic,” U.S. Patent Number 4,587,627 (1986).
8. [8] Itoh, T. and Tsujii, S., “Structure of Parallel Multipliers for a Class of Fields GF(2m),” Information and Computation, Vol. 83, pp. 21
   40 (1989).
9. [9] Hasan, M. A., Wang, M. and Bhargava, V. K., “Modular Construction of Low Complexity Parallel Multipliers for a Class of Finite Fields GF(2m),” IEEE Trans. Computers, Vol. 41, pp. 962
   971 (1992).
10. [10] Wu, H., Hasan, M. A. and Blake, I. F., “New Lowcomplexity Bit-parallel Finite Field Multipliers Using Eeakly Dual Bases,” IEEE Trans. Computers, Vol. 47, pp. 1223
    1234 (1998).
11. [11] Wu, H. and Hasan, M. A., “Low-complexity Bitparallel Multipliers for a Class of Finite Fields,” IEEE Trans. Computers, Vol. 47, pp. 883
    887 (1998).
12. [12] Lee, C.-Y., Lu, E.-H. and Lee, J.-Y., “Bit-parallel Systolic Multipliers for GF(2m) Fields Defined by All-one and Equally Spaced Polynomials,” IEEE Trans. Computers, Vol. 50, pp. 385
    393 (2001).
13. [13] Sunar, B. and Koç, C. K., “Mastrovito multiplier for all trinomials,” IEEE Trans. Computers, Vol. 48, pp. 522
    527 (1999).
14. [14] Wu, H., Hasan, M. A. and Blake, I. F., “On Complexity of Bit-parallel Finite Field Multiplier,” Proc. Canadian Workshop Information Theory’97 (1997).
15. [15] Wu, H. “Bit-parallel Finite Field Multiplier and Square Using Polynomial Basis,” IEEE Trans. Computers, Vol.51, pp. 750
    758 (2002).
16. [16] Chiou, C. W. “Concurrent Error Detection in Array Multipliers for GF(2m) Fields,” Electronics Letters, Vol. 38, pp. 688
    689 (2002).