Jaw-Kuen Shiau This email address is being protected from spambots. You need JavaScript enabled to view it.¹ and Ching-En Tseng¹

¹Department of Aerospace Engineering, Tamkang University Tamsui, Taiwan 251, R.O.C

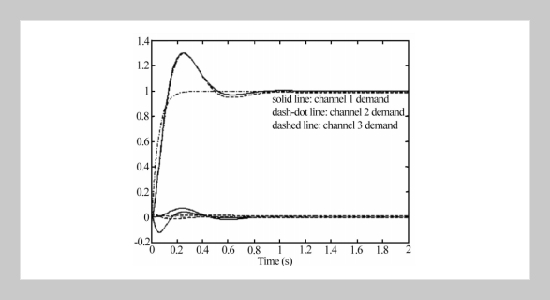
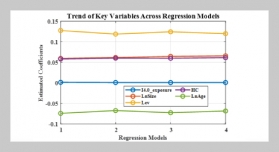
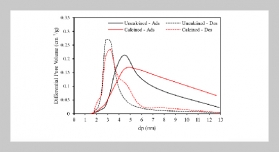
REFERENCES

1. [1] Zhou, K. and Doyle, J. C., Essentials of Robust Control, Prentice Hall, NJ, U.S.A., (1998).
2. [2] Doyle, J. C., Glover, K., Khargonekar, P. P. and Francis, B. A., “State-Space Solutions to Standard H2 and H
   Control Problems,” IEEE Trans. Automat. Contr., Vol. 34, pp. 831
   847 (1989).
3. [3] Grigoriadis, K. M. and Skelton, R. E., “Fixed-Order Controller Problems Using Alternating Projection Methods,” Proc. 1994 CDC, pp. 2003
   2008 (1994).
4. [4] Gahinet, P. and Apkarian, P., “A Linear Matrix Inequality Approach to H
   Control,” Int. J. Robust and Nonlinear Control, Vol. 4, pp. 421
   448 (1994).
5. [5] Ghaoui, L. El. and Balkrishnan, V., “Synthesis of Fixed-Structure Controllers via Numerical Optimization,” Proc. 1994 CDC, pp. 2678
   2683 (1994).
6. [6] Shiau, J. K. and Chow, J. H., “Robust Decentralized State Feedback Controller Design Using an Iterative Linear Matrix Inequality Algorithm,” Preprints of 13th IFAC World Congress, Vol. H, pp. 203
   208 (1996).
7. [7] Shiau, J. K. and Chow, J. H., “Structurally Constrained Suboptimal Control Design Using an Iterative Linear Matrix Inequality Algorithm Based on a Dual Design Formulation”, Tamkang Journal of Science and Engineering, Vol. 1, pp. 133
   143 (1998).
8. [8] Wang, S. and Chow, J. H., “Coprime Factors, Strictly Positive Real Functions, LMI and Low-Order Controller Design For SISO Systems”, Proceedings of the 37th IEEE Conference on Decision and Control, Tampa, FL, U.S.A., Dec., pp. 2738
   2744 (1998).
9. [9] Wang, S. and Chow, J. H., “Low-Order Controller Design for Model-Matching Optimization Using Coprime Factors and Linear Matrix Inequalities,” Proceeding of American Control Conference 1999, Vol. 3, pp. 1871
   1875, (1999).
10. [10] Shiau, J. K., “Coprime Factors, Outer Functions, Linear Matrix Inequalities and Low-order Controller Design for Discrete Single-Input-Single-Output Systems,” 2000 Automatic Control Conference, Taiwan, pp. 291
    296 (2000).
11. [11] Shiau, J. K. and Chung, Y. H., “Robust Low-Order Controller Design for Model-Matching Optimization Using Coprime Factors for Linear Discrete MIMO Systems”, JSME International Journal, Series C, Vol. 46, pp. 713
    719 (2003).
12. [12] Gahinet, P., Nemirovskii, A., Laub, A. J. and Chilali, M., LMI Control Toolbox, The MathWorks, Inc., (1995).
13. [13] Vidyasagar, M., Control System Synthesis: A Factorization Approach, MIT Press, Cambridge, MA, U.S.A. (1985).
14. [14] Maciejowski, J. M., Multivariable Feedback Design, Addison Wesley, NY, U.S.A. (1989).