Fu-Cheng Yang This email address is being protected from spambots. You need JavaScript enabled to view it.1, Sheau-Wen Shiah2 , Tzeng-Yuan Heh2 , Chan-Yung Jen3 and Wen-I Chang4 1Graduate School of Defense Science, Chung Cheng Institute of Technology, National Defense University, Taoyuan, Taiwan 335, R.O.C.
2Department of Power Vehicle and System Engineering, Chung Cheng Institute of Technology, National Defense University, Taoyuan, Taiwan 335, R.O.C.
3Department of Mechanical and Marine Engineering, Naval Academy, Kaohsiung, Taiwan 813, R.O.C.
4Duo De Company of Limited Liability, Taoyuan, Taiwan 325, R.O.C.
Received:
April 11, 2007
Accepted:
August 29, 2007
Publication Date:
June 1, 2008
Download Citation:
||https://doi.org/10.6180/jase.2008.11.2.06
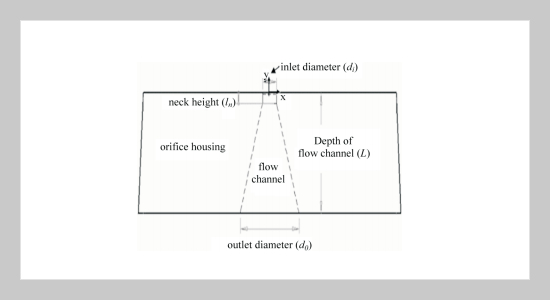
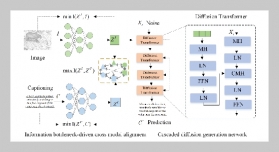
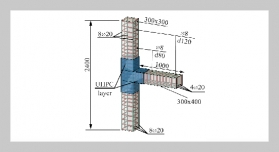
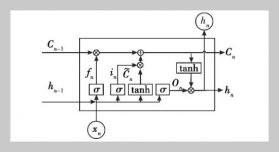
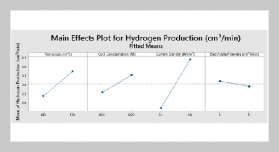
The purpose of this paper is using numerical simulation method to discuss the influences of high-velocity waterjet on different types of orifice which is made of diamond. Not only we aim at establishing the model of analysis to the orifice geometry but also change the design conditions of the orifices in order to find the optimal orifice shape. During the numerical simulation, a two-dimensional axial-symmetric model is established first, and then some practical geometric parameters are considered for the orifice, including flow channel with 0.5 mm in depth, 0.1 mm to 0.56 mm for the diameters of inlet and outlet, and 0.005 mm to 0.346 mm for the neck height. The analytic results can show the pressure and velocity distributions of high-velocity waterjets in the orifices under different conditions and can be used for assessment of orifice geometric design. Through this study, it is expected to know more physical insight about the effect of high-velocity waterjet on orifice geometry and further to improve the life cycle of orifice.ABSTRACT
Keywords:
Design of Orifices, High-Velocity Waterjets, Numerical Simulation
REFERENCES