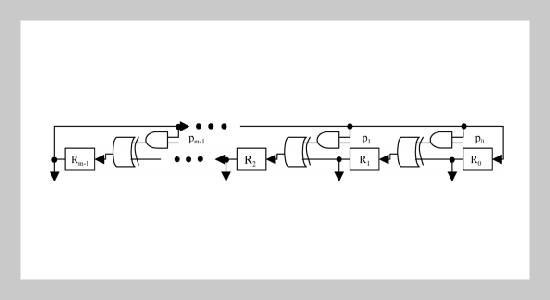
REFERENCES
- [1] Macwilliams, P. J. and Sloane, N. J. A., The Theory of Error-Correcting Codes, Amsterdam: North-Holland (1978).
- [2] Lidl., R. and Niederreiter, H., Introduction to Finite Fields and Their Applications, New York: Cambridge Univ. Press (1994).
- [3] Blahut, R. E., Fast Algorithms for Digital Signal Processing, Reading, Mass.: Addison-Wesley (1985).
- [4] Reed, I. S. and Truong, T. K., “The Use of Finite Fields to Compute Convolutions,” IEEE Trans. Information Theory, Vol. IT-21, pp. 208�213 (1975).
- [5] Benjauthrit, B. and Reed, I. S., “Galois Switching Functions and Their Applications,” IEEE Trans. Computers, Vol. C-25, pp. 78�86 (1976).
- [6] Wang, C. C. and Pei, D., “A VLSI Design for Computing Exponentiation in GF(2m) and its Application to Generate Pseudorandom Number Sequences,” IEEE Trans. Computers, Vol. 39, pp. 258�262 (1990).
- [7] Massey, J. L. and Omura, J. K., “Computational Method and Apparatus for Finite Field Arithmetic,” U.S. Patent Number 4,587,627, May (1986).
- [8] Wang, C. C. et al., “VLSI Architectures for Computing Multiplications and Inverses in GF(2m),” IEEE Trans. Computers, Vol. C-34, pp. 709�717 (1985).
- [9] Reyhani-Masoleh, A. and Hasan, M. A., “A New Construction of Massey-Omura Parallel Multiplier over GF(2m),” IEEE Trans. Computers, Vol. 51, pp. 511� 520 (2002).
- [10] Lee, C. Y. and Chiou, C. W., “Efficient Design of Low-Complexity Bit-Parallel Systolic Hankel Multipliers to Implement Multiplication in Normal and Dual Bases of GF(2m),” IEICE Transactions on Fundamentals of Electronics, Communications and Computer Science, Vol. E88-A, pp. 3169�3179 (2005).
- [11] Chiou, C. W. and Lee, C. Y., “Multiplexer-Based Double-Exponentiation for Normal Basis of GF (2m),” Computers & Security, Vol. 24, pp. 83�86 (2005).
- [12] Lee, C. Y., Chen, Y. H., Chiou, C. W. and Lin, J. M., “Unified Parallel Systolic Multipliers over GF(2m),” Journal of Computer Science and Technology, Vol. 22, pp. 28�38 (2007).
- [13] Berlekamp, E. R., “Bit-Serial Reed-Solomon Encoders,” IEEE Trans. Information Theory, Vol. IT-28, pp. 869�874 (1982).
- [14] Wu, H., Hasan, M. A. and Blake, I. F., “New LowComplexity Bit-Parallel Finite Field Multipliers Using Weakly Dual Bases,” IEEE Trans. Computers, Vol. 47, pp. 1223�1234 (1998).
- [15] Wu, H. and Hasan, M. A., “Low Complexity Bit-Parallel Multipliers for a Class of Finite Fields,” IEEE Trans. Computers, Vol. 47, pp. 883�887 (1998).
- [16] Bartee, T. C. and Schneider, D. J., “Computation with Finite Fields,” Information and Computing, Vol. 6, pp. 79�98 (1963).
- [17] Laws, B. A. J. and Rushforth, C. K., “A Cellular-Array Multiplier for GF(2m),” IEEE Trans. Computers, Vol. C-20, pp. 1573�1578 (1971).
- [18] Yeh, C. S., Reed, I. S. and Truong, T. K., “Systolic Multipliers for Finite Fields GF(2m),” IEEE Trans. Computers, Vol. C-33, pp. 357�360 (1984).
- [19] Mastrovito, E. D., “VLSI Architectures for Multiplication Over Finite Field GF(2m), Applied Algebra, Algebraic Algorithms, and Error-Correcting Codes,” Proc. Sixth Int’l Conf., AAECC-6, T. Mora, ed., Rome, pp. 297�309 (1988).
- [20] Mastrovito, E. D., “VLSI Architectures for Computations in Galois Fields,” PhD thesis, Linkoping Univ., Dept. of Electrical Eng., Linkoping, Sweden (1991).
- [21] Koç, Ç. K. and Sunar, B., “Low-Complexity Bit-Parallel Canonical and Normal Basis Multipliers for a Class of Finite Fields,” IEEE Trans. Computers, Vol. 47, pp. 353�356 (1998).
- [22] Sunar, B. and Koç, Ç. K., “Mastrovito Multiplier for All Trinomials,” IEEE Trans. Computers, Vol. 48, pp. 522�527 (1999).
- [23] Elia, M., Leone, M. and Visentin, C., “Low Complexity Bit-Parallel Multipliers for GF(2m) with Generator Polynomial xm+xk +1,” Electronics Letters, Vol. 35, pp. 551�552 (1999).
- [24] Wu, H., “Bit-Parallel Finite Field Multiplier and Square Using Polynomial Basis,” IEEE Trans. Computers, Vol. 51, pp. 750�758 (2002).
- [25] Lee, C. Y., “Low Complexity Bit-Parallel Systolic Multiplier Over GF(2m) Using Irreducible Trinomials,” IEE Proc.-Comput. Digit. Tech., Vol. 150, pp. 39�42 (2003).
- [26] Chiou, C. W., Lin, L. C., Chou, F. H. and Shu, S. F., “Low Complexity Finite Field Multiplier Using Irreducible Trinomials,” IEE Electronics Letters, Vol. 39, pp. 1709�1711 (2003).
- [27] Itoh, T. and Tsujii, S., “Structure of Parallel Multipliers for a Class of Fields GF(2m),” Information and Computation, Vol. 83, pp. 21�40 (1989).
- [28] Lee, C. Y., Lu, E. H. and Lee, J. Y., “Bit-Parallel Systolic Multipliers for GF(2m) Fields Defined by All-One and Equally-Spaced Polynomials,” IEEE Trans. Computers, Vol. 50, pp. 385�393 (2001).
- [29] Hasan, M., Wang, M. and Bhargava, V. K., “Modular Construction of Low Complexity Parallel Multipliers for a Class of Finite Fields GF(2m),” IEEE Trans. Computers, Vol. 41, pp. 962�971 (1992).
- [30] Paar, C., “A New Architecture for a Parallel Finite Field Multiplier with Low Complexity Based on Composite Fields,” IEEE Trans. Computers, Vol. 45, pp. 856�861 (1996).
- [31] Paar, C., Fleischmann, P. and Roelse, P., “Efficient Multiplier Architectures for Galois Fields GF(24n),” IEEE Trans. Computers, Vol. 47, pp. 162�170 (1998).
- [32] Chiou, C. W., Lee, C. Y. and Lin, J. M., “Efficient Systolic Arrays for Power-Sum, Inversion, and Division in GF(2m),” International Journal of Computer Sciences and Engineering Systems, Vol. 1, pp. 27�41 (2007).
- [33] Lee, C. Y., Lin, J. M. and Chiou, C. W., “Scalable and Systolic Architecture for Computing Double Exponentiation Over GF(2m),” Acta Applicandae Mathematicae, Vol. 93, pp. 161�178 (2006).
- [34] Lee, C. Y., Chiou, C. W., Deng, A. W. and Lin, J. M., “Low-Complexity Bit-Parallel Systolic Architectures for Computing A(x)B2 (x) Over GF(2m),” IEE Proceedings-Circuits, Devices and Systems, Vol. 153, pp. 399�406 (2006).
- [35] Lee, C. Y., Chen, Y. H., Chiou, C. W. and Lin, J. M., “Unified Parallel Systolic Multipliers over GF(2m),” Journal of Computer Science and Technology, Vol. 22, pp. 28�38 (2007).