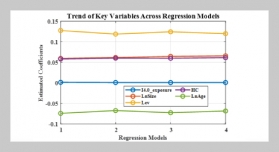
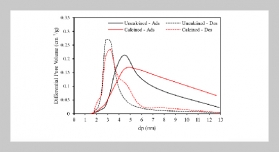
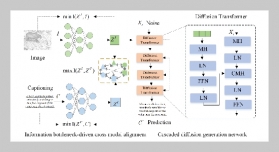
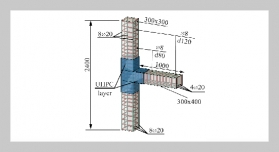
REFERENCES
- [1] Chen, S. L. and Liu, C. L., “The Optimal Consignment Policy for the Manufacturer under Supply Chain Coordination,” International Journal of Production Research, Vol. 46, pp. 5121�5143 (2008).
- [2] Goyal, S. K., “An Integrated Inventory Model for a Single Supplier-Single Customer Problem,” International Journal of Production Research, Vol. 15, pp. 107�111 (1976).
- [3] Lu, L., “A One-Vendor Multi-Buyer Integrated Inventory Model,” European Journal of Operational Research, Vol. 81, pp. 312�323 (1995).
- [4] Hill, R, M., “The Single-Vendor Single-Buyer Integrated Production Inventory Model with a Generalized Policy,” European Journal of Operational Research, Vol. 97, pp. 493�499 (1997).
- [5] Goyal, S. K. and Nebebe, F., “Determination of Economic Production-Shipment Policy for a SingleVendor-Single-Buyer System,” International Journal of Production Research, Vol.121, pp.175�178 (2000).
- [6] Goyal, S. K., “On Improving the Single-Vendor Single-Buyer Integrated Production Inventory Model with a Generalize Policy,” European Journal of Operation Research, Vol. 125, pp. 429�430 (2000).
- [7] Seifert, R. W., Thonemann, U. W. and Hausman, W. H., “Optimal Procurement Strategies for Online Spot Markets,” European Journal of Operational Research, Vol. 152, pp. 781�799 (2004).
- [8] Haksoz, C. and Seshadri, S., “Supply Chain Operations in the Presence for a Spot Market: A Review with Discussion,” Journal of the Operational Research Society, Vol. 58, pp. 1412�1429 (2007).
- [9] Chen, S. L. and Liu, C. L., “Procurement Strategies in the Presence of the Spot Market � An Analytical Framework,” Production Planning & Control, Vol. 18, pp. 297�309 (2007).
- [10] Li, J. and Liu, L., “Supply Chain Coordination with Manufacturer’s Limited Reserve Capacity: An Extended Newsboy Problem,” International Journal of Production Economics, Vol. 112, pp. 860�868 (2008).
- [11] Darwish, M. A., “Economic Selection of Process Mean for Single-Vendor Single-Buyer Supply Chain,” European Journal of Operational Research, Vol. 199, pp. 162�169 (2009).
- [12] Chen, S. L. and Huang, S. C., “Managing Supply Chain Risk with Options and Online Spot Markets,” Journal of Statistics & Management Systems, Vol. 13, pp. 389�407 (2010).
- [13] Arshinder, Kanda, A. and Deshmukh, S. G., “Supply Chain Coordination: Perspectives, Empirical Studies and Research Directions,” International Journal of Production Economics, Vol. 115, pp. 316�335 (2008).
- [14] Sana, S. S., Goyal, S. K. and Chaudhuri, K., “On a Volume Flexible Inventory Model for Items with an Imperfect Production System,” International Journal of Production Research, Vol. 2, pp. 64�80 (2007a).
- [15] Sana, S. S., Goyal, S. K. and Chaudhuri, K., “An Imperfect Production Process in a Volume Flexible Inventory Model,” International Journal of Production Economics, Vol. 105, pp. 548�559 (2007b).
- [16] Sana, S. S., “An Economic Production Lot Size Model in an Imperfect Production System,” European Journal of Operational Research, Vol. 200, pp. 158�170 (2010a).
- [17] Sana, S. S., “A Production-Inventory Model in an Imperfect Production Process,” European Journal of Operational Research, Vol. 200, pp. 451�464 (2010b).
- [18] Sana, S. S., “Demand Influenced by Enterprises’ Initiatives --- a Multi-Item EOQ Model of Deteriorating and Ameliorating Items,” Mathematical and Computer Modelling, Vol. 52, pp. 284�302 (2010c).
- [19] Sana, S. S., “Optimal Selling Price and Lot Size with Time Varying Deteriorating and Partial Backlogging,” Applied Mathematics and Computation, Vol. 217, pp. 185�194 (2010d).
- [20] Taguchi, G., Introduction to Quality Engineering, Tokyo: Asian Productivity Organization (1986).