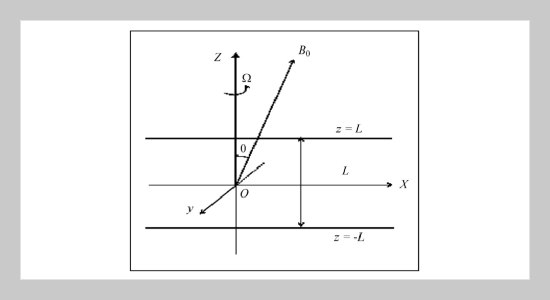
REFERENCES
- [1] Seth, G. S. and Ghosh, S. K., “Hydromagnetic Flow in a Rotating Channel with Hall Effects,” Acta Ciencia Indica, Vol. XXV M, No. 4, pp. 403�406 (1999).
- [2] Ghosh, S. K., “Hall Effect on Unsteady Hydromagnetic Flow in a Rotating Channel Permeated by an Inclined Magnetic Field in the Presence of an Oscillator,” Czechoslovak J. Physics, Vol. 49, No. 4, pp. 465�472 (1999a). doi: 10.1007/BF01692243
- [3] Ghosh, S. K., “Hall Effect on Unsteady Hydromagnetic Flow in a Rotating Channel Permeated by an Inclined Magnetic Field in the Presence of an Oscillator (Erratum),” Czechoslovak Journal of Physics, Vol. 49, No. 12, p. 1751 (1999b). doi: 10.1007/BF01692243
- [4] Ghosh, S. K. and Pop, I., “Hall Effects on MHD Plasma Couette Flow in a Rotating Environment,” International Journal of Applied Mechanics and Engineering, Vol. 9, No. 2, pp. 293�305 (2004).
- [5] Ghosh, S. K. and Pop, I., “Comments on MHD Plasma Flow in a Rotating Environment in the Presence of an Inclined Magnetic Field,” International Journal of Applied Mechanics and Engineering, Vol. 9, No. 4, pp. 821�826 (2004).
- [6] Mondal, G., Mandal, K. K. and Choudhury, G., “Hall Effects on MHD Plasma Couette Flow in a Rotating System,” Journal of Physical Society of Japan, Vol. 51, p. 2010 (1982). doi: 10.1143/JPSJ.52.470
- [7] Sato, H., “The Hall Effects in the Viscous Flow of Ionized Gas between Parallel Plates under Transverse Magnetic Field,” Journal of Physical Society of Japan, Vol. 16, No. 7, pp. 1427�1433 (1961). doi: 10.1143/ JPSJ.16.1427
- [8] Guria, M., Das, S., Jana, R. N. and Ghosh, S. K., “Oscillatory Couette Flow in the Presence of an Inclined Magnetic Field,” Meccanica, Vol. 44, pp. 555�564 (2009). doi: 10.1007/s11012-009-9195-1
- [9] Seth, G. S., Nandkeolyar, R., Mahto, N. and Singh, S. K., “MHD Couette Flow in a Rotating System in the Presence of Inclined Magnetic Field,” Applled Mathematical Science, Vol. 3, No. 59, pp. 2919�2932 (2009).
- [10] Seth, G. S. and Ghosh, S. K., “Unsteady Hydromagnetic Flow in a Rotating Channel in the Presence of Oblique Magnetic Field,” Int. J. Engng. Sci., Vol. 24, p. 1183 (1986). doi: 10.1016/0020-7225(86)90013-3
- [11] Seth, G. S. and Ghosh, S. K., “Hydromagnetic Flow in a Rotating Channel in the Presence of Inclined Magnetic Field,” Proceeding of the Mathematical Society Banaras Hindu University, Vol. 11, p. 111 (1995). doi: 10.1007/BF01692243
- [12] Ghosh, S. K. and Bhattacharjee, P. K., “Hall Effects on Steady Hydromagnetic Flow in a Rotating Channel in the Presence of an Inclined Magnetic Field,” Czechoslovak Journal of Physics, Vol. 50, p. 759 (2000).
- [13] Seth, G. S., Ansary, M. S., Mahto, N. and Singh, S. K., “Hydromagnetic Flow in a Rotating Channel in the Presence of an Inclined Magnetic Field,” Acta Ciencia Indica, Vol. XXXIV M, No. 3, pp. 1279�1288 (2008).
- [14] Seth, G. S., Nandkeolyar, R. and Ansari, M. S., “Hall Effects on Oscillatory Hydromagnetic Couette Flow in a Rotating System,” International Journal of Academic Research, Vol. 1, pp. 6�17 (2009).
- [15] Das, S., Sarkar, B. C. and Jana, R. N., “Hall Effects on MHD Couette Flow in Rotating System,” International Journal of Computer Application, Vol. 35, No. 13, pp. 22�30 (2011).
- [16] Bég, O. A., Sim, L., Zueco, J. and Bhargava, R., “Numerical Study of Magnetohydrodynamic Viscous Plasma Flow in Rotating Porous Media with Hall Currents and Inclined Magnetic Field Influence,” Commun Nonlinear Sci Numer Simulat, Vol. 15, pp. 345� 359 (2010). doi: 10.1016/j.cnsns.2009.04.008
- [17] Seth, G. S., Mahato, G. K. and Nandkeolyar, R., “Effects of Hall Current on Oscillatory Hydromagnetic Couette Flow of Class-II in a Rotating System in the Presence of an Inclined Magnetic Field,” International Journal of Advanced Scientific and Technical Research, Vol. 1, No. 1, pp. 31�47 (2011).
- [18] Seth, G. S., Nandkeolyar, R. and Ansari, M. S., “Effects of Hall Current and Rotation on Unsteady MHD Couette Flow in the Presence of an Inclined Magnetic Field,” Journal of Appllied Fluid Mechanics, Vol. 5, No. 2, pp. 67�74 (2012).
- [19] Ghosh, S. K. and Pop, I., “An Analytical Approach to MHD Plasma Behavior of a Rotating Environment in the Presence of an Inclined Magnetic Field as Compared to Excitation Frequency,” International Journal of Applied Mechanics and Engineering, Vol. 11, No. 4, pp. 845�856 (2006).
- [20] Cowling, T. G., Magnetohydrodynamics, Intersscience, New York (1957). doi: 10.1063/1.3060498