Free Convection Heat Transfer from a Non-Isothermal Permeable Cone with Suction and Temperature-Dependent Viscosity

Ching-Yang Cheng

doi:10.6180/jase.2015.18.1.03

Free Convection Heat Transfer from a Non-Isothermal Permeable Cone with Suction and Temperature-Dependent Viscosity

Mechanical Engineering

Ching-Yang Cheng This email address is being protected from spambots. You need JavaScript enabled to view it.¹

¹Department of Mechanical Engineering, Southern Taiwan University of Science and Technology, Tainan, Taiwan 710, R.O.C.

Received: December 2, 2014
Accepted: January 12, 2015
Publication Date: March 1, 2015

Download Citation: ||https://doi.org/10.6180/jase.2015.18.1.03

ABSTRACT

This work studies the free convection heat transfer from a non-isothermal permeable cone with suction and temperature-dependent viscosity. A suitable coordinate transformation is used to transform the governing equations into nonsimilar boundary layer solutions, and the cubic spline collocation method is then employed to solve the obtained governing equations. The local Nusselt number is presented as functions of suction variables for different values of viscosity-variation parameter, surface temperature exponent, and Prandtl number. Results show that an increase in the suction variable or the viscosity-variation parameter tends to increase the local Nusselt number of the vertical permeable cones with temperature-dependent viscosity. The effect of the viscosity-variation parameter on the local Nusselt number is significant only for small values of suction variables.

Keywords: Free Convection, Heat Transfer, Vertical Permeable Cone, Temperature-Dependent Viscosity

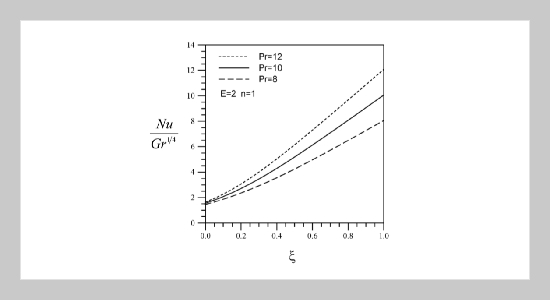
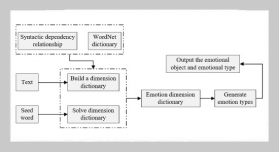
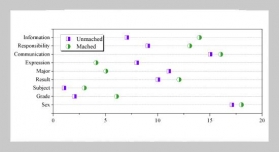
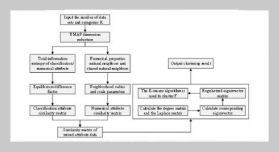
REFERENCES

[1] Gray, J., Kassory, D. R. and Tadjeran, H., “The Effect of Significant Viscosity Variation on Convective Heat Transport in Water-Saturated Porous Media,” J. Fluid Mech., Vol. 117, pp. 233 249 (1982). doi: 10.1017/ S0022112082001608
[2] Lings, J. X. and Dybbs, A., “Forced Convection Over a Flat Plate Submersed in a Porous Medium: Variable Viscosity Case,” Paper 87-WA/HT-23, ASME, New York (1987).
[3] Kafoussius, N. G. and Williams, E. M., “The Effect of Temperature Dependent Viscosity on the Free Convective Laminar Boundary Layer Flow Past a Vertical Isothermal Plate,” Acta Mech., Vol. 110, pp. 123 137 (1995). doi: 10.1007/BF01215420
[4] Kafoussius, N. G. and Rees, D. A. S., “Numerical Study of the Combined Free and Forced Convective Laminar Boundary Layer Flow Past a Vertical Isothermal Flat Plate with Temperature Dependent Viscosity,” Acta Mech., Vol. 127, pp. 39 50 (1998). doi: 10.1007/ BF01170361
[5] Molla, M. M., Hossain, M. A. and Gorla, R. S. R., “Natural Convection Flow from an Isothermal Circular Cylinder with Temperature Dependent Viscosity,” Heat Mass Transfer, Vol. 41, pp. 594 598 (2005). doi: 10.1007/s00231-004-0576-7
[6] Cheng, C. Y., “Nonsimilar Boundary Layer Analysis of Double-Diffusive Convection from a Vertical Truncated Cone in a Porous Medium with Variable Viscosity,” Appl. Math. Comput., Vol. 212, pp. 185 193 (2009). doi: 10.1016/j.amc.2009.02.012
[7] Pal, D. and Mondal, H., “Influence of TemperatureDependent Viscosity and Thermal Radiation on MHDForced Convection over a Non-Isothermal Wedge,” Appl. Math. Comput., Vol. 212, pp. 194 208 (2009). doi: 10.1016/j.amc.2009.02.013
[8] Cheng, C. Y., “Natural Convection Boundary Layer Flow of Fluid with Temperature-Dependent Viscosity from a Horizontal Cylinder with Constant Surface Heat Flux,” Appl. Math. Comput., Vol. 217, pp. 83 91 (2010). doi: 10.1016/j.amc.2010.05.011
[9] Hering, R. G. and Grosh, R. J., “Laminar Free Convectionfrom a Non-Isothermal Cone,” Int. J. Heat Mass Transfer, Vol. 5, pp. 1059 1068 (1962). doi: 10. 1016/0017-9310(62)90059-5
[10] Na, T. Y. and Chiou, J. P., “Laminar Natural Convection Over a Frustum of a Cone,” Appl. Sci. Res.,Vol. 35, pp. 409 421 (1979). doi: 10.1007/BF00420389
[11] Yih, K. A., “Effect of Radiation on Natural Convection about a Truncated Cone,” Int. J. Heat Mass Transfer, Vol. 42, pp. 4299 4305 (1999). doi: 10.1016/S0017- 9310(99)00092-7
[12] Hossain, M. A. and Paul, S. C., “Free Convection from a Vertical Permeable Circular Cone with Non-Uniform Surface Temperature,” Acta Mech., Vol. 151, pp. 103 114 (2001). doi: 10.1007/BF01272528
[13] Chamkha, A. J., “Coupled Heat and Mass Transfer by Natural Convection about a Truncated Cone in the Presence of Magnetic Field and Radiation Effects,” Num. Heat Transfer, Part A: Appl., Vol. 39, pp. 511 530 (2001). doi: 10.1080/10407780120202
[14] Postelnicu, A., “Free Convection about a Vertical Frustum of a Cone in a MicropolarFluid,” Int. J. Eng. Sci., Vol. 44, pp. 672 682 (2006). doi: 10.1016/j.ijengsci. 2005.10.009
[15] Cheng, C. Y., “Natural Convection of a Micropolar Fluid from a Vertical Truncated Cone with Power-Law Variation in Surface Temperature,” Int. Comm. Heat Mass Transfer, Vol. 35, pp. 39 46 (2008). doi: 10. 1016/j.icheatmasstransfer.2007.05.018
[16] Cheng, C. Y., “Natural Convection Boundary Layer Flow of a Micropolar Fluid Over a Vertical Permeable Cone with Variable Wall Temperature,” Int. Comm. Heat Mass Transfer, Vol. 38, pp. 429 433 (2011). doi: 10.1016/j.icheatmasstransfer.2010.12.021
[17] Cheng, C. Y., “Free Convective Boundary-Layer Flow Over a Vertical Truncated Cone in a Bidisperse Porous Medium,” Proc. of the World Congress on Engineering 2013, London, U.K., July 3 5, pp. 1997 2002 (2013).
[18] Rubin, S. G. and Graves, R. A., “Viscous Flow Solution with a Cubic Spline Approximation,” Comput. Fluids, Vol. 3, pp. 1 36 (1975). doi: 10.1016/0045- 7930(75)90006-7
[19] Wang, P. and Kahawita, R., “Numerical Integration of a Partial Differential Equations Using Cubic Spline,” Int. J. Comput. Math.,Vol. 13, pp. 271 286 (1983). doi: 10.1080/00207168308803369