REFERENCES
- [1] DeSanto, J. A., “Exact Spectral Formalism for Rough surface Scattering,” Journal of the Optical Society of America A, Vol. 2, pp. 2202�2207 (1985). doi: 10. 1364/JOSAA.2.002202
- [2] Wombell, R. J. and DeSanto, J. A., “The Reconstruction of Shallow Rough-surface Profiles from Scattered field Data,” Inverse Problems, Vol. 7, No. 1 (1991). doi: 10.1088/0266-5611/7/1/002
- [3] Li, Q., Chan, H. and Tsang, L., “Monte Carlo Simulations of Wave Scattering from Lossy Dielectric Random Rough Surfaces Using the Physics-based Twogrid Method and the Canonical-grid Method,” IEEE Transactions on Antennas and Propagation, Vol. 47, No. 4, pp. 752�763 (1999). doi: 10.1109/8.768816
- [4] Guo, L. X. and Wu, Z. S., “Moment Method with Wavelet Expansions for Fractal Rough Surface Scattering,” Chinese Physics Letters, Vol. 19, No. 11 (2002). doi: 10.1088/0256-307X/19/11/315
- [5] Altuncu, Y., Yapar, A. and Akduman, I., “Numerical Computation of the Green’s Function of a Layered Media with Rough Interfaces,” Microw. Opt. Technol. Lett., Vol. 49, No. 5, pp. 1204�1209 (2007). doi: 10. 1002/mop.22401
- [6] Yildiz, S., Altuncu, Y., Yapar, A. and Akduman, I., “On the Scattering of Electromagnetic Waves by Periodic Rough Dielectric Surfaces: a Boa Solution,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 46, No. 9, pp. 2599�2606 (2008). doi: 10.1109/TGRS. 2008.919275
- [7] Tezel, N. S., “Electromagnetic Scattering from Inhomogeneous Impedance Periodic Rough Surfaces by Transform Technique,” Microwave and Optical Technology Letters, Vol. 51, No. 1, pp. 116�119 (2009). doi: 10.1002/mop.23954
- [8] Boix, R. R., Fructos, A. L. and Mesa, F., “Closed-form Uniform Asymptotic Expansions of Green’sFunctions in Layered Media,” IEEE Trans. Antennas Propag., Vol. 58, No. 9, pp. 2934–2945 (2010). doi: 10.1109/ TAP.2010.2052569
- [9] Zamani, H., Tavakoli, A. and Dehmollaian, M., “Scattering from Layered Rough Surfaces: Analytical and Numerical Investigations,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 54, pp. 3685�3696 (2016). doi: 10.1109/TGRS.2016.2524639
- [10] Yapar, A., Ozdemir, O., Sahinturk, H. and Akduman, I., “A Newton Method for the Reconstruction of Perfectly Conducting Slightly Rough Surface Profiles,” IEEE Transactions on Antennas and Propagation, Vol. 54, No. 1, pp. 275�279 (2006). doi: 10.1109/TAP.2005. 861513
- [11] Akduman, I., Kress, R. and Yapar, A., “Iterative Reconstruction of Dielectric Rough Surface Profiles at Fixed Frequency,” Inverse Problems, Vol. 22, No. 3 (2006). doi: 10.1088/0266-5611/22/3/013
- [12] Gürbüz, T., Aslanyürek, B., Karabulut, E. P. and Akduman, I., “An Efficient Non linear Imaging Approach for Dielectric Objects Buried under a Rough Surface,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 52, No. 5, pp. 3013�3022 (2014). doi: 10.1109/TGRS.2013.2268662
- [13] Shamsaddini, M., Tavakoli, A. and Dehkhoda, P., “Inverse Electromagnetic Scattering of a Dielectric Cylinder Buried below a Slightly Rough Surface Using a New Intelligence Approach,” Iranian Conference on Electrical Engineering (ICEE), May (2015). doi: 10. 1109/IranianCEE.2015.7146246
- [14] Roy, D. G. and Mudaliar, S., “Domain Derivatives in Dielectric Rough Surface Scattering,” IEEE Transactions on Antennas and Propagation, Vol. 63, pp. 4486� 4495 (2015). doi: 10.1109/TAP.2015.2463682
- [15] Van Den Berg, P. M. and Kleinman, R. E., “A Contrast Source Inversion Method,” Inverse Problems, Vol. 13, No. 6, pp. 1607–1620 (1997). doi: 10.1088/0266-5611/ 13/6/013
- [16] Van Den Berg, P. M., Van Broekhoven, A. and Abubakar A., “Extended Contrast Source Inversion,” Inverse Problems, Vol. 15, No. 5, pp. 1325–1344 (1999). doi: 10.1088/0266-5611/15/5/315
- [17] Altuncu, Y., Yapar, A. and Akduman, I., “Numerical Computation of the Green’s Function of a Layered Media with Rough Interface,” Microw. Opt. Technol. Lett., Vol. 49, No. 5, pp. 1204–1209 (2007). doi: 10. 1002/mop.22401
- [18] Xu, K., Zhong, Y., Song, R., Chen, X. and Ran, L., “Multiplicative-regularized FFT-twofold Subspace-based Optimization Method for Inverse Scattering Problems,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 53, pp. 841�850 (2015). doi: 10.1109/TGRS. 2014.2329032
- [19] Ye, X., Zhong, Y. and Chen, X., “Reconstructing Perfectly Electric Conductors by the Subspace-based Optimization Method with Continuous Variables,” Inverse Problems, Vol. 27, No. 5, pp. 1�14 (2011). doi: 10. 1088/0266-5611/27/5/055011
- [20] Li, C. L., Chien, W., Huang, C. H. and Chiu, C. C., “Time Domain Microwave Imaging for a Buried Dielectric Cylinder by Dynamic Differential Evolution,” International Journal of Applied Electromagnetics and Mechanics, Vol. 34, No. 1, 2, pp. 73�86 (2010). (EI) (SCI).
- [21] Wan, Y., Yu, C. Y., Sun, C. H. and Chiu, C. C., “The Reconstruction of Time Domain Through-wall Imaging for a Metallic Cylinder,” Imaging Science Journal, Vol. 63, No. 2, pp. 81�84 (2015). (EI) (SCI). doi: 10.1179/1743131X14Y.0000000084
- [22] Lee, Y. H., Cheng, Y. T., Chiu, C. C. and Chang, S. P., “Microwave Imaging for Half-space Imperfect Conductors,” Nondestructive Testing and Evaluation, Vol. 30, No. 1, pp. 49�62 (2015). (EI) (SCI). doi: 10.1080/ 10589759.2014.992430
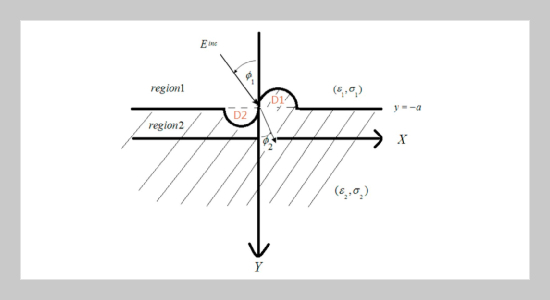
- [23] Wang, X., Gan, Y. B. and Li, L. W., “Electromagnetic Scattering by Partially Buried PEC Cylinder at the Dielectric Rough Surface Interface: TMCase,”IEEE Antennas and Wireless Propagation Letters,Vol.2(2003). doi: 10.1109/LAWP.2003.822200
- [24] Chen, X., “The Role of Regularization Parameter of Subspace-based Optimization Method in Solving Inverse Scattering Problems,” IEEE Microwave Conference on AsiaPacific, pp. 1549�1552, 7�10 Dec. (2009). doi: 10.1109/APMC.2009.5384411
- [25] Habashy, T. M., Oristaglio, M. L. and De Hoop, A. T., “Simultaneous Nonlinear Reconstruction of 2-dimensional Permittivity and Conductivity,” Radio Science, Vol. 29, pp. 1101�1118 (1994). doi: 10.1029/93RS03448
- [26] Press, W. H., Teukolsky, S. A., Vetterling, W. T. and Flannery, B. P., Numerical Recipes in FORTRAN, Second Edition, The Press Syndicate of the University of Cambridge (1992).
- [27] Chen, X., “Subspace-based Optimization Method for Solving Inverse Scattering Problems,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 48, pp. 42�49 (2010). doi: 10.1109/TGRS.2009.2025122
- [28] Storn, R. and Price, K., “Differential Evolution - a Simple and Efficient Adaptive Scheme for Global Optimization over Continuous Spaces,” Technical Report TR-95-012, International Computer Science Institute,
Berkeley (1995).
- [29] Sun, C. H. and Chiu, C. C., “Inverse Scattering of Dielectric Cylindrical Target Using Dynamic Differential Evolution and Self-adaptive Dynamic Differential Evolution,” International Journal of RF and Microwave Computer-Aided Engineering, Vol. 23, No. 5, pp. 579– 585 (2013). doi: 10.1002/mmce.20692
- [30] Chiu, C. C., Sun, C. H., Li, C. L. and Huang, C. H., “Comparative Study of Some Population-based Optimization Algorithms on Inverse Scattering of a Two dimensional Perfectly Conducting Cylinder in Slab Medium,” IEEE Transactions on Geoscience and Remote Sensing, Vol. 51, pp. 2302–2315 (2013). doi: 10.1109/ TGRS.2012.2208756