M. A. Moelyadi This email address is being protected from spambots. You need JavaScript enabled to view it.1, Y. A. T. Rohmahwati1 and A. P. Nugraha1 1Flight Physics Research Group, Faculty of Mechanical and Aerospace Engineering, Institut Teknologi Bandung, Jl. Ganesa 10 Bandung 40132, Indonesia
Received:
November 2, 2017
Accepted:
April 16, 2018
Publication Date:
June 1, 2019
Download Citation:
||https://doi.org/10.6180/jase.201906_22(2).0007
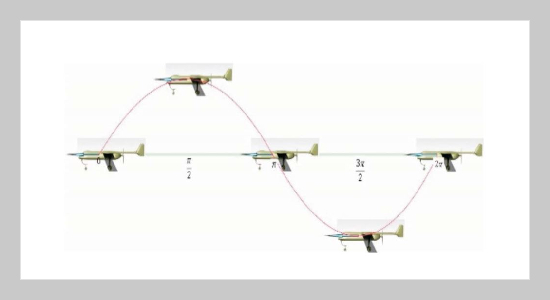
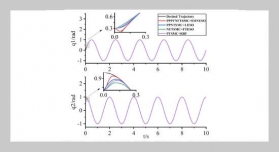
Static and dynamic stability derivatives known as aero model are important numbers used in preliminary design of a vehicle and often used in engineering flight simulator. These measure how much change in forces and moments acting on the vehicle when there is a small change in flight condition parameters such as angle of attack, altitude and speed. Increasing the capability of computational fluid dynamics (CFD) method in solving complex unsteady flow around complex geometry, it can be used for determining dynamic stability derivatives of the vehicle which was often conducted in wind tunnel requiring higher cost and quite complex mechanism measurement device. The paper presents the determination of longitudinal static and dynamic stability derivatives of the designed UAV using computational fluid dynamics method and Fourier analysis. For obtaining dynamic stability derivatives, the UAV model have to move in either the single periodic vertical movement or single pitch rotation or in both combination of them with sines or cosines function. The unsteady flow solution around the moving vehicle are then obtained by solving Reynolds averaged navier-stokes equations. The results of unsteady aerodynamic forces and moments of the UAV are then analyzed using Fourier method to yield static and dynamic stability derivatives. Based on the results show that the designed UAV has good longitudinal dynamic stability agreement compared to vortex Lattice method. It fulfills the requirement for the UAV to have longitudinal stability in terms of pitching moment with the rate of angle of attack and pitch rate.ABSTRACT
Keywords:
Unmanned Aerial Vehicle, Longitudinal Dynamic Stability Derivatives, Unsteady Aerodynamics, Computational Fluid Dynamics, Fourier Analysis
REFERENCES
doi: 10.2514/6.2017-3791