P. Sirish Kumar This email address is being protected from spambots. You need JavaScript enabled to view it.1 and V.B.S. Srilatha Indira Dutt2 1Department of Electronics Communication Engineering, Aditya Institute of Technology and Management, Tekkali 532201, India.
2Department of Electronics Communication Engineering, GITAM University, Visakhapatnam 530045, India.
Received:
August 19, 2020
Accepted:
September 4, 2020
Publication Date:
February 1, 2021
Download Citation:
||https://doi.org/10.6180/jase.202102_24(1).0009
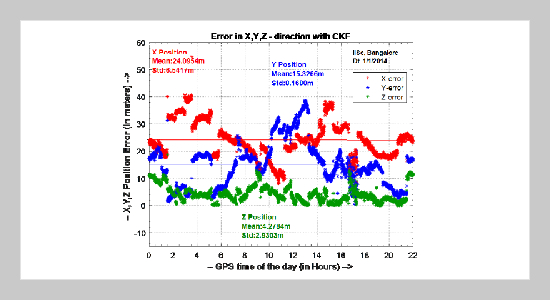
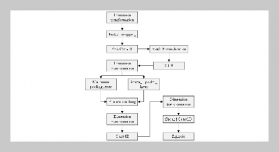
Some study methods, like least-squares, Kalman filter, are worked out so far to minimize this error factor and improve the GPS positioning accuracy. This paper presents a fast, accurate, and a new method in implementing Kalman Filter for GPS positioning, based on the correntropy criterion designated as the Correntropy Kalman Filter (CKF). The suggested model is evaluated using numerous 2-Dimensional and 3-Dimensional accuracy metrics in X, Y, Z directions. The proposed method results show that the positioning accuracy for all three co-ordinates is up to 34 %, significantly greater than the general approach (Traditional Kalman Filter).ABSTRACT
Keywords:
Accuracy; Correntropy; Correntropy Kalman Filter; Global Positioning System; Kalman Filter
REFERENCES