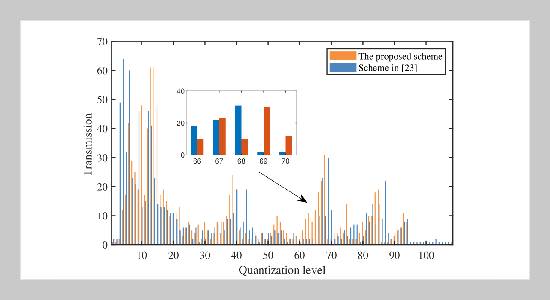
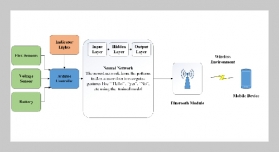
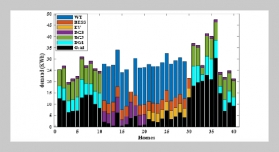
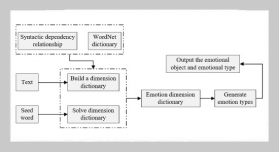
- [1] X. Chu and M. Li, (2018) “Event-triggered fault estimation and sliding mode fault-tolerant control for a class of nonlinear networked control systems" Journal of the Franklin Institute 355(13): 5475–5502.
- [2] C. Peng, J. Zhang, and Q.-L. Han, (2018) “Consensus of multiagent systems with nonlinear dynamics using an integrated sampled-data-based event-triggered communication scheme" IEEE Transactions on Systems, Man, and Cybernetics: Systems 49(3): 589–599.
- [3] H. Liang, L. Chen, Y. Pan, and H.-K. Lam, (2022) “Fuzzy-based robust precision consensus tracking for uncertain networked systems with cooperative-antagonistic interactions" IEEE Transactions on Fuzzy Systems:
- [4] Z. Cao, B. Niu, G. Zong, and N. Xu, (2023) “Smallgain technique-based adaptive output constrained control design of switched networked nonlinear systems via event-triggered communications" Nonlinear Analysis: Hybrid Systems 47: 101299.
- [5] X. Liu, J. Zhang, and Y. Xia, (2019) “Co-design of quantization and event-driven control for networked control systems" IEEE Transactions on Systems, Man, and Cybernetics: Systems 51(5): 3103–3110.
- [6] L. Cao, Y. Pan, H. Liang, and T. Huang, (2022) “Observer-Based Dynamic Event-Triggered Control for Multiagent Systems With Time-Varying Delay" IEEE Transactions on Cybernetics:
- [7] F. Cheng, H. Wang, L. Zhang, A. Ahmad, and N. Xu, (2022) “Decentralized adaptive neural two-bit-triggered control for nonstrict-feedback nonlinear systems with actuator failures" Neurocomputing 500: 856–867.
- [8] L. Xing, C. Wen, H. Su, J. Cai, and L. Wang, (2015) “A new adaptive control scheme for uncertain nonlinear systems with quantized input signal" Journal of the Franklin Institute 352(12): 5599–5610.
- [9] S. Song, J. H. Park, B. Zhang, and X. Song, (2021) “Composite adaptive fuzzy finite-time quantized control for full state-constrained nonlinear systems and its application" IEEE Transactions on Systems, Man, and Cybernetics: Systems 52(4): 2479–2490.
- [10] X. Song, M. Wang, C. K. Ahn, and S. Song, (2021) “Finite-time fuzzy bounded control for semilinear PDE systems with quantized measurements and markov jump actuator failures" IEEE Transactions on Cybernetics 52(7): 5732–5743.
- [11] B. Xuhui, W. Taihua, H. Zhongsheng, and C. Ronghu, (2015) “Iterative learning control for discrete-time systems with quantised measurements" IET Control Theory & Applications 9(9): 1455–1460.
- [12] X. Cai, K. Shi, S. Zhong, and X. Pang, (2021) “Dissipative sampled-data control for high-speed train systems with quantized measurements" IEEE Transactions on Intelligent Transportation Systems 23(6): 5314–5325.
- [13] D. Shen and C. Zhang, (2020) “Zero-error tracking control under unified quantized iterative learning framework via encoding–decoding method" IEEE Transactions on Cybernetics 52(4): 1979–1991.
- [14] X. Chu and M. Li, (2019) “Integrated event-triggered fault estimation and fault-tolerant control for discretetime fuzzy systems with input quantization and incomplete measurements" Journal of the Franklin Institute 356(13): 7112–7143.
- [15] S. Ha, L. Chen, H. Liu, and S. Zhang, (2022) “Command filtered adaptive fuzzy control of fractional-order nonlinear systems" European Journal of Control 63: 48–60.
- [16] H. Qiu, H. Liu, and X. Zhang, (2022) “Composite adaptive fuzzy backstepping control of uncertain fractionalorder nonlinear systems with quantized input" International Journal of Machine Learning and Cybernetics: 1–15. DOI: 10.1007/s13042-022-01666-9.
- [17] Y. Xu, D. Shen, and X. Bu, (2017) “Zero-error convergence of iterative learning control using quantized error information" IMA Journal of Mathematical Control and Information 34(3): 1061–1077.
- [18] D. Shen and Y. Xu, (2016) “Iterative learning control for discrete-time stochastic systems with quantized information" IEEE/CAA Journal of Automatica Sinica 3(1): 59–67.
- [19] S. Lee and H.-J. Song, (2021) “Accurate statistical model of radiation patterns in analog beamforming including random error, quantization error, and mutual coupling" IEEE Transactions on Antennas and Propagation 69(7): 3886–3898.
- [20] S. Liu, B. Niu, G. Zong, X. Zhao, and N. Xu, (2022) “Data-driven-based event-triggered optimal control of unknown nonlinear systems with input constraints" Nonlinear Dynamics 109(2): 891–909.
- [21] Z. Hou and S. Jin, (2010) “A novel data-driven control approach for a class of discrete-time nonlinear systems" IEEE Transactions on Control Systems Technology 19(6): 1549–1558.
- [22] Y. Zhao, X. Liu, H. Yu, and J. Yu, (2020) “Model-free adaptive discrete-time integral terminal sliding mode control for PMSM drive system with disturbance observer" IET Electric Power Applications 14(10): 1756–1765.
- [23] X. Bu, Y. Qiao, Z. Hou, and J. Yang, (2018) “Model free adaptive control for a class of nonlinear systems using quantized information" Asian Journal of Control 20(2): 962–968.
- [24] H. Zhang, J. Zhou, Q. Sun, J. M. Guerrero, and D. Ma, (2015) “Data-driven control for interlinked AC/DC microgrids via model-free adaptive control and dual-droop control" IEEE Transactions on Smart Grid 8(2): 557–571.
- [25] Y. Weng and X. Gao, (2016) “Data-driven robust output tracking control for gas collector pressure system of coke ovens" IEEE Transactions on Industrial Electronics 64(5): 4187–4198.
- [26] S. Zhang, P. Zhou, Y. Xie, and T. Chai, (2022) “Improved model-free adaptive predictive control method for direct data-driven control of a wastewater treatment process with high performance" Journal of Process Control 110: 11–23.
- [27] D. Xu, Y. Shi, and Z. Ji, (2017) “Model-free adaptive discrete-time integral sliding-mode-constrained-control for autonomous 4WMV parking systems" IEEE Transactions on Industrial Electronics 65(1): 834–843.
- [28] X. Li, C. Ren, S. Ma, and X. Zhu, (2020) “Compensated model-free adaptive tracking control scheme for autonomous underwater vehicles via extended state observer" Ocean Engineering 217: 107976.
- [29] X. Bu, P. Zhu, Q. Yu, Z. Hou, and J. Liang, (2020) “Model-free adaptive control for a class of nonlinear systems with uniform quantizer" International Journal of Robust and Nonlinear Control 30(16): 6383–6398. [30] H. Zhao, L. Peng, and H. Yu, (2022) “Quantized modelfree adaptive iterative learning bipartite consensus tracking for unknown nonlinear multi-agent systems" Applied Mathematics and Computation 412: 126582.