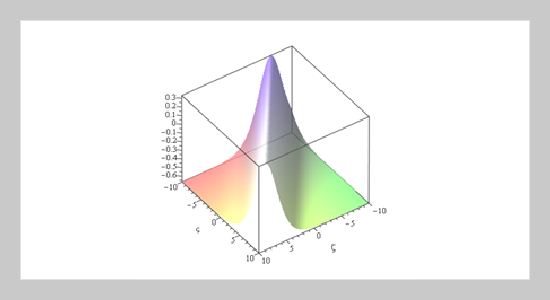
- [1] M. Inc, H. Rezazadeh, J. Vahidi, M. Eslami, M. A. Akinlar, M. N. Ali, and Y.-M. Chu, (2020) “New soli�tary wave solutions for the conformable Klein-Gordon equation with quantic nonlinearity" Aims Math 5(6): 6972–6984.
- [2] D. Kumar and G. C. Paul, (2021) “Solitary and periodic wave solutions to the family of nonlinear conformable frac�tional Boussinesq-like equations" Mathematical Meth�ods in the Applied Sciences 44(4): 3138–3158.
- [3] N. H. M. Shahen, M. H. Bashar, and M. S. Ali, (2020) “Dynamical analysis of long-wave phenomena for the nonlinear conformable space-time fractional (2+ 1)- dimensional AKNS equation in water wave mechanics" Heliyon 6(10):
- [4] D. Kumar, G. C. Paul, A. R. Seadawy, and M. T. Darvishi, (2022) “A variety of novel closed-form soli�ton solutions to the family of Boussinesq-like equations with different types" Journal of Ocean Engineering and Science 7(6): 543–554.
- [5] N. Raza, A. R. Seadawy, S. Arshed, and M. H. Rafiq, (2022) “A variety of soliton solutions for the Mikhailov�Novikov-Wang dynamical equation via three analytical methods" Journal of Geometry and Physics 176: 104515.
- [6] H. Rezazadeh, (2018) “New solitons solutions of the complex Ginzburg-Landau equation with Kerr law non�linearity" Optik 167: 218–227.
- [7] R. Saleh, S. M. Mabrouk, and A. M. Wazwaz, (2021) “The singular manifold method for a class of fractional�order diffusion equations" Waves in Random and Com�plex Media: 1–12.
- [8] H. M. Baskonus and H. Bulut, (2016) “Exponential prototype structures for (2+ 1)-dimensional Boiti-Leon�Pempinelli systems in mathematical physics" Waves in Random and Complex Media 26(2): 189–196.
- [9] M. S. M. Shehata, H. Rezazadeh, E. H. M. Zahran, E. Tala-Tebue, and A. Bekir, (2019) “New optical soliton solutions of the perturbed Fokas-Lenells equation" Com�munications in Theoretical Physics 71(11): 1275.
- [10] K. U. Tariq, A. R. Seadawy, and M. Younis, (2018) “Ex�plicit, periodic and dispersive optical soliton solutions to the generalized nonlinear Schrödinger dynamical equation with higher order dispersion and cubic-quintic nonlinear terms" Optical and Quantum Electronics 50: 1–19.
- [11] R. A. Attia, D. Lu, and M. M. Khater, (2018) “Structure of new solitary solutions for the Schwarzian Korteweg De Vries equation and (2+ 1)-Ablowitz-Kaup-Newell-Segur equation" Phys. J 1(3): 234–254.
- [12] M. K. Elboree, (2015) “Derivation of soliton solutions to nonlinear evolution equations using He’s variational principle" Applied Mathematical Modelling 39(14): 4196–4201.
- [13] A. Biswas, M. O. Al-Amr, H. Rezazadeh, M. Mirza�zadeh, M. Eslami, Q. Zhou, S. P. Moshokoa, and M. Belic, (2018) “Resonant optical solitons with dual-power law nonlinearity and fractional temporal evolution" Op�tik 165: 233–239.
- [14] R. Cimpoiasu, (2020) “Multiple invariant solutions of the 3 D potential Yu–Toda–Sasa–Fukuyama equation via symmetry technique" International Journal of Mod�ern Physics B 34(20): 2050188.
- [15] B. Ghanbari, K. S. Nisar, and M. Aldhaifallah, (2020) “Abundant solitary wave solutions to an extended nonlin�ear Schrödinger’s equation with conformable derivative using an efficient integration method" Advances in Dif�ference Equations 2020(1): 1–25.
- [16] M. Eslami and H. Rezazadeh, (2016) “The first integral method for Wu–Zhang system with conformable time�fractional derivative" Calcolo 53(3): 475–485.
- [17] K. A. Muhamad, T. Tanriverdi, A. A. Mahmud, and H. M. Baskonus, (2023) “Interaction characteristics of the Riemann wave propagation in the (2+ 1)-dimensional generalized breaking soliton system" International Jour�nal of Computer Mathematics 100(6): 1340–1355.
- [18] H. F. Ismael, H. M. Baskonus, H. Bulut, and W. Gao, (2023) “Instability modulation and novel optical soli�ton solutions to the Gerdjikov–Ivanov equation with M�fractional" Optical and Quantum Electronics 55(4): 303.
- [19] N. Raza, F. Salman, A. R. Butt, and M. L. Gandarias, (2023) “Lie symmetry analysis, soliton solutions and qual�itative analysis concerning to the generalized q-deformed Sinh-Gordon equation" Communications in Nonlin�ear Science and Numerical Simulation 116: 106824.
- [20] N. Raza, S. Arshed, F. Salman, J. F. Gómez-Aguilar, and J. Torres-Jiménez, (2022) “Phase characterization and new optical solitons of a pulse passing through nonlin�ear dispersive media" Modern Physics Letters B 36(19): 2250098.
- [21] N. Raza, A. Batool, and M. Inc, (2022) “New hyper�bolic and rational form solutions of (2+ 1)-dimensional generalized Korteweg-de Vries model" Journal of Ocean Engineering and Science:
- [22] N. Raza, M. H. Rafiq, A. Bekir, and H. Rezazadeh, (2022) “Optical solitons related to (2+ 1)-dimensional Kundu–Mukherjee–Naskar model using an innovative integration architecture" Journal of Nonlinear Optical Physics & Materials 31(03): 2250014.
- [23] J. Manafian and M. Lakestani, (2016) “Applica�tion of tan (ϕ/2)-expansion method for solving the Biswas–Milovic equation for Kerr law nonlinearity" Op�tik 127(4): 2040–2054.
- [24] U. Younas, J. Ren, L. Akinyemi, and H. Rezazadeh, (2023) “On the multiple explicit exact solutions to the double-chain DNA dynamical system" Mathematical Methods in the Applied Sciences 46(6): 6309–6323.
- [25] T. A. Sulaiman, H. Bulut, and H. M. Baskonus. “Con�struction of various soliton solutions via the sim�plified extended sinh-Gordon equation expansion method”. In: ITM Web of Conferences. 22. EDP Sci�ences, 2018, 1062.
- [26] N. Salamat, A. H. Arif, M. Mustahsan, M. M. S. Mis�sen, and V. B. S. Prasath, (2022) “On compacton trav�eling wave solutions of Zakharov-Kuznetsov-Benjamin�Bona-Mahony (ZK-BBM) equation" Computational and Applied Mathematics 41(8): 365.
- [27] N. Raza and A. Zubair, (2019) “Optical dark and singular solitons of generalized nonlinear Schrödinger’s equation with anti-cubic law of nonlinearity" Modern Physics Letters B 33(13): 1950158.
- [28] N. Raza, A. R. Seadawy, M. Kaplan, and A. R. Butt, (2021) “Symbolic computation and sensitivity analysis of nonlinear Kudryashov’s dynamical equation with applica�tions" Physica Scripta 96(10): 105216.
- [29] A. A. Mahmud, T. Tanriverdi, and K. A. Muhamad, (2023) “Exact traveling wave solutions for (2+ 1)- dimensional Konopelchenko-Dubrovsky equation by using the hyperbolic trigonometric functions methods" Inter�national Journal of Mathematics and Computer in Engineering: DOI: 10.2478/ijmce-2023-0002.
- [30] A.-M. Wazwaz, (2005) “Compact and noncompact physi�cal structures for the ZK–BBM equation" Applied Math�ematics and Computation 169(1): 713–725.
- [31] A.-M. Wazwaz, (2008) “The extended tanh method for new compact and noncompact solutions for the KP–BBM and the ZK–BBM equations" Chaos, Solitons & Frac�tals 38(5): 1505–1516.
- [32] S. Bibi and S. T. Mohyud-Din, (2014) “Traveling wave solutions of ZK-BBM equation sine-cosine method" Com�mun. Numer. Anal. 2014: 1–9.
- [33] H. Naher, (2015) “New approach of (G’/G)-expansion method and new approach of generalized (G’/G)-expansion method for ZKBBM equation" Journal of the Egyptian Mathematical Society 23(1): 42–48.
- [34] A. Ali, M. A. Iqbal, and S. T. Mohyud�Din, (2016) “Solitary wave solutions Za�kharov–Kuznetsov–Benjamin–Bona–Mahony (ZK–BBM) equation" Journal of the Egyptian Mathematical Society 24(1): 44–48.
- [35] J. Zhao and W. Li, (2014) “Exact solitary wave solu�tion in the ZK-BBM equation" Journal of Nonlinear Dynamics 2014:
- [36] M. Song and Z. Liu, (2014) “Periodic wave solutions and their limits for the ZK–BBM equation" Applied Mathematics and Computation 232: 9–26.
- [37] M. Song and C. Yang, (2010) “Exact traveling wave solutions of the Zakharov-Kuznetsov–Benjamin-Bona�Mahony equation" Applied Mathematics and Com�putation 216(11): 3234–3243.
- [38] Ö. Güner, A. Bekir, L. Moraru, and A. Biswas. “Bright and dark soliton solutions of the generalized Za�kharov–Kuznetsov–Benjamin–Bona–Mahony nonlin�ear evolution equation”. In: Proc. Rom. Acad. Ser. A. 16. 3. 2015, 422–429.
- [39] R. Kumar, M. Kumar, and A. Kumar, (2013) “Some soliton solutions of non linear partial differential equations by Tan-Cot method" IOSR Journal of Mathematics (IOSR-JM) 6(6): 23–28.
- [40] M. Kayum, R. Roy, M. A. Akbar, and M. S. Osman, (2021) “Study of W-shaped, V-shaped, and other type of surfaces of the ZK-BBM and GZD-BBM equations" Optical and Quantum Electronics 53(7): 1–20.
- [41] O. Tasbozan and A. Kurt, (2015) “Approximate analyt�ical solution of ZK-BBM equation" Sohag Journal of Mathematics 2(2): 57–60.
- [42] M. M. Kabir, A. Khajeh, E. Abdi Aghdam, and A. Yousefi Koma, (2011) “Modified Kudryashov method for finding exact solitary wave solutions of higher-order nonlinear equations" Mathematical methods in the Applied Sciences 34(2): 213–219.
- [43] S. M. Mirhosseini-Alizamini, N. Ullah, J. Sabi’u, H. Rezazadeh, and M. Inc, (2021) “New exact solutions for nonlinear Atangana conformable Boussinesq-like equa�tions by new Kudryashov method" International Jour�nal of Modern Physics B 35(12): 215016.