Hazem Ali Attia This email address is being protected from spambots. You need JavaScript enabled to view it.1 1Department of Mathematics, College of Science, Al-Qasseem University, P.O. Box 237, Buraidah 81999, KSA
Received:
October 25, 2004
Accepted:
March 12, 2005
Publication Date:
June 1, 2009
Download Citation:
||https://doi.org/10.6180/jase.2009.12.2.14
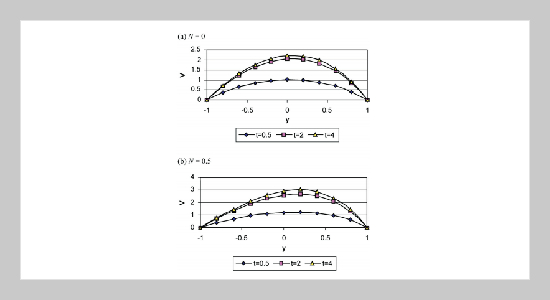
The unsteady Poiseuille flow of a viscoelastic fluid between two horizontal porous plates in the presence of a porous medium is studied with heat transfer. The fluid viscosity is assumed to be temperature dependent and the fluid is subjected to a uniform suction from above and injection from below. The plates are maintained at two fixed but different temperatures. The motion of the fluid is produced by a uniform horizontal constant pressure gradient. The equation of motion and the energy equation are solved numerically to yield the velocity and temperature distributions.ABSTRACT
Keywords:
Non-Newtonian Fluid, Heat Transfer, Variable Properties, Numerical Solution, Porous Medium
REFERENCES