Kuo-Ching Chang1, Chui-Liang Chiang2 and Chung-Bow Lee This email address is being protected from spambots. You need JavaScript enabled to view it.1 1Department of Applied Mathematics, National Chung Hsing University, Taichung, Taiwan 402, R.O.C.
2Department of Food Science and Technology, Central Taiwan University of Science and Technology, Taichung, Taiwan 406, R.O.C.
Received:
December 30, 2010
Accepted:
June 23, 2011
Publication Date:
March 1, 2012
Download Citation:
||https://doi.org/10.6180/jase.2012.15.1.02
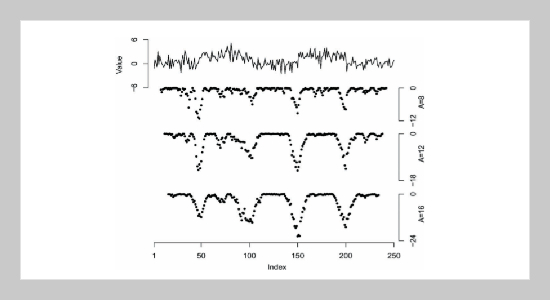
A fast two-stage (TS) algorithm by window method is proposed. First, we apply the window method by using the log-likelihood ratio measure to find a subset of candidate change-points; and use dynamic programming (DP) algorithm on the chosen subset to obtain good initial change-points which will be proximate to the locations of the true change-points. Secondary, the segmental K-means (SKM) algorithm is applied on the initial change-points obtained in the first stage. Some simulated data sets are investigated for four algorithms and the results show that our algorithm works very well. In the comparison of CPU times, our TS algorithm is fast and can be up to 19.07 times than the speed of DP algorithm.ABSTRACT
Keywords:
Change-Points, Exponential Family, Dynamic Programming, Segmental K-Means
REFERENCES