Arvind Kumar This email address is being protected from spambots. You need JavaScript enabled to view it.¹

¹Department of Mathematics, I.K. Gujral Punjab Technical University, Jalandhar, Punjab, India

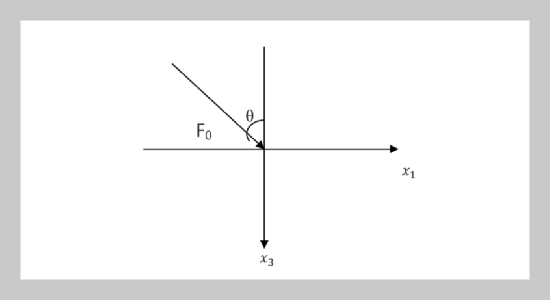
REFERENCES

1. [1] Eringen, A. C., “Theory of Thermomicrostretch Elastic Solids,” International Journal of Engineering Science, Vol. 28, No. 12, pp. 1291–1301 (1990).
2. [2] Cicco, S. D., “Stress Concentration Effects in Microstretch Elastic Bodies,” International Journal of Engineering Sciences, Vol. 41, pp. 187199 (2003).
3. [3] Ciarletta, M. and Scalia, A., “Some Results in Linear Theory of Thermomicrostretch Elastic Solids,” Meccanica, Vol. 39, pp. 191–206 (2004).
4. [4] Iesan, D. and Quintanilla, R., “Thermal Stresses in Microstretch Elastic Plates,” Int. J. Eng. Sci., Vol. 43, pp. 885–907 (2005). doi: 10.1016/j.ijengsci.2005.03.005
5. [5] Aouadi, M., “Thermomechanical Interactions in a Generalized Thermomicrostretch Elastic Half Space,” Journal of Thermal Stresses, Vol. 29, pp. 511528 (2006). doi: 10.1080/01495730500373495
6. [6] Marin, M., “A Domain of Influence Theorem for Microstretch Elastic Materials,” Nonlinear Analysis, Real World Applications, Vol. 11, pp. 34463452 (2010). doi: 10.1016/j.nonrwa.2009.12.005
7. [7] Marin, M., “Apartition of Energy in Thermoelasticity of Microstretch Bodies,” Nonlinear Anal., Real World Appl., Vol. 11, pp. 2436–2447 (2010a). doi: 10.1016/ j.nonrwa.2009.07.014
8. [8] Marin, M., “Lagrange Identity Method for Microstretch Thermoelastic Materials,” J. Math. Anal. Appl., Vol. 363, pp. 275–286 (2010b). doi: 10.1016/j.jmaa. 2009.08.045
9. [9] Passarella, F. and Tibullo, V., “Some Results in Linear Theory of Thermoelasticity Backward in Time for Microstretch Materials,” J. Therm. Stress, Vol. 33, pp. 559–576 (2010). doi: 10.1080/01495731003772811
10. [10] Kumar, R. and Kumar, A., “Elastodynamic Response Due to Mechanical Forces in a Microstretch Thermoelastic Medium with Mass Diffusion,” Material Physics and Mechanics, Vol. 22, pp. 4452 (2015).
11. [11] Kumar, R., Kumar, A. and Singh, D., “Thermomechanical Interactions of Laser Pulse with Microstretch Thermoelastic Medium,” Archives of Mechanics, Vol. 67, No. 6, pp. 439456 (2015).
12. [12] Gad, N. S., “Effects of Hall Currents on Peristaltic Transport with Compliant Walls,” Applied Mathematics and Computation, Vol. 235, pp. 546554 (2014). doi: 10.1016/j.amc.2014.02.081
13. [13] Ezzat, M. A. and Awad, E. S., “Micropolar Generalized Magneto-thermoelasticity with Modified Ohm’s and Fourier’s Laws,” J. Math. Anal. Appl., Vol. 353, pp. 99113 (2009). doi: 10.1016/j.jmaa.2008.11.058
14. [14] Zakaria, M., “Effects of Hall Current and Rotationon Magneto-micropolar Generalized Thermoelasticity Due to Ramp-type Heating,” International Journal of Electromagnetics and Applications, Vol. 2, No. 3, pp. 24 32 (2012). doi: 10.5923/j.ijea.20120203.02
15. [15] Eringen, A. C., Microcontinuum Field Theories I: Foundations and Solids, Springer-Verleg, New York (1999).
16. [16] Eringen, A. C., “Plane Waves in Non-local Micropolar Elasticity,” Int. J. Eng. Sci., Vol. 22, pp. 1113–1121 (1984). doi: 10.1016/0020-7225(84)90112-5
17. [17] Dhaliwal, R. S. and Singh, A., Dynamic Coupled Thermoelasticity, Hindustan Publication Corporation, New Delhi (1980).