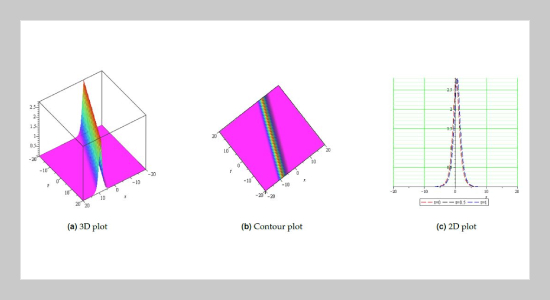
- [1] H. Qin, M. Khater, and R. A. Attia, (2020) “Inelastic interaction and blowup new solutions of nonlinear and dispersive long gravity waves" Journal of Function Spaces 2020: DOI: 10.1155/2020/5362989.
- [2] D. Kumar and M. Kaplan, (2018) “New analytical solutions of (2+ 1)-dimensional conformable time fractional Zoomeron equation via two distinct techniques" Chinese journal of physics 56(5): 2173–2185. DOI: 10.1016/j.cjph.2018.09.013.
- [3] K. Hosseini, A. Korkmaz, A. Bekir, F. Samadani, A. Zabihi, and M. Topsakal, (2021) “New wave form solutions of nonlinear conformable time-fractional Zoomeron equation in (2+ 1)-dimensions" Waves in Random and Complex Media 31(2): 228–238. DOI: 10.1080/17455030.2019.1579393.
- [4] H. Rezazadeh, (2018) “New solitons solutions of the complex Ginzburg-Landau equation with Kerr law nonlinearity" Optik 167: 218–227. DOI: 10.1016/j.ijleo.2018.04.026.
- [5] H. Ahmad, M. N. Alam, and M. Omri, (2021) “New computational results for a prototype of an excitable system" Results in Physics 28: 104666. DOI: 10.1016/j.rinp.2021.104666.
- [6] S.-W. Yao, L. Akinyemi, M. Mirzazadeh, M. Inc, K. Hosseini, and M. ¸Senol, (2021) “Dynamics of optical solitons in higher-order Sasa–Satsuma equation" Results in Physics 30: 104825. DOI: 10.1016/j.rinp.2021.104825.
- [7] M. Hashemi, (2021) “A novel approach to find exact solutions of fractional evolution equations with non-singular kernel derivative" Chaos, Solitons & Fractals 152:111367. DOI: 10.1016/j.chaos.2021.111367.
- [8] A. Yusuf, T. A. Sulaiman, M. Inc, S. Abdel-Khalek, and K. Mahmoud, (2021) “M- truncated optical soliton and their characteristics to a nonlinear equation governing the certain instabilities of modulated wave trains":
- [9] H. U. Rehman, N. Ullah, and M. Imran, (2021) “Exact solutions of Kudryashov–Sinelshchikov equation using two analytical techniques" The European Physical Journal Plus 136(6): 1–13.
- [10] M. Nuruzzaman, D. Kumar, and G. C. Paul, (2021) “Fractional low-pass electrical transmission line model: Dynamic behaviors of exact solutions with the impact of fractionality and free parameters" Results in Physics 27: 104457.
- [11] A. Yoku¸s, H. Durur, T. A. Nofal, H. Abu-Zinadah, M. Tuz, and H. Ahmad, (2020) “Study on the applications of two analytical methods for the construction of traveling wave solutions of the modified equal width equation" Open Physics 18(1): 1003–1010.
- [12] R. Cimpoiasu, (2020) “Multiple invariant solutions of the 3 D potential Yu–Toda–Sasa–Fukuyama equation via symmetry technique" International Journal of Modern Physics B 34(20): 2050188.
- [13] N. A. Kudryashov, (2012) “One method for finding exact solutions of nonlinear differential equations" Communications in Nonlinear Science and Numerical Simulation 17(6): 2248–2253.
- [14] N. A. Kudryashov, (2020) “Method for finding highly dispersive optical solitons of nonlinear differential equations" Optik 206: 163550.
- [15] N. A. Kudryashov, (2020) “Solitary wave solutions of hierarchy with non-local nonlinearity" Applied Mathematics Letters 103: 106155.
- [16] N. A. Kudryashov and E. V. Antonova, (2020) “Solitary waves of equation for propagation pulse with power nonlinearities" Optik 217: 164881.
- [17] N. A. Kudryashov, D. V. Safonova, and A. Biswas, (2019) “Painlevé Analysis and a Solution to the Traveling Wave Reduction of the Radhakrishnan—Kundu—Lakshmanan Equation" Regular and Chaotic Dynamics 24(6): 607–614.
- [18] N. A. Kudryashov, (2022) “Exact solutions of the complex Ginzburg–Landau equation with law of four powers of nonlinearity" Optik 265: 169548.
- [19] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2015) “Exact solutions for the fractional differential equations by using the first integral method" Nonlinear engineering 4(1): 15–22.
- [20] H. Rezazadeh, D. Kumar, T. A. Sulaiman, and H. Bulut, (2019) “New complex hyperbolic and trigonometric solutions for the generalized conformable fractional Gardner equation" Modern Physics Letters B 33(17):1950196.
- [21] M. S. Shehata, H. Rezazadeh, E. H. Zahran, E. Tala-Tebue, and A. Bekir, (2019) “New optical soliton solutions of the perturbed Fokas-Lenells equation" Communications in Theoretical Physics 71(11): 1275.
- [22] H. Aminikhah, A. H. R. Sheikhani, and H. Rezazadeh, (2016) “Travelling wave solutions of nonlinear systems of PDEs by using the functional variable method" Boletim da sociedade paranaense de matemática 34(2): 213–229.
- [23] M. Eslami and H. Rezazadeh, (2016) “The first integral method for Wu–Zhang system with conformable timefractional derivative" Calcolo 53(3): 475–485.
- [24] G.Wang, K. Yang, H. Gu, F. Guan, and A. Kara, (2020) “A (2+ 1)-dimensional sine-Gordon and sinh-Gordon equations with symmetries and kink wave solutions" Nuclear Physics B 953: 114956.
- [25] G.Wang, (2021) “A new (3+ 1)-dimensional Schrödinger equation: derivation, soliton solutions and conservation laws" Nonlinear Dynamics 104(2): 1595–1602.
- [26] G. Wang, (2021) “A novel (3+ 1)-dimensional sine-Gorden and a sinh-Gorden equation: derivation, symmetries and conservation laws" Applied Mathematics Letters 113: 106768.
- [27] G. Wang, (2021) “Symmetry analysis, analytical solutions and conservation laws of a generalized KdV–Burgers–Kuramoto equation and its fractional version"Fractals 29(04): 2150101.
- [28] G. Wang and A.-M. Wazwaz, (2022) “On the modified Gardner type equation and its time fractional form" Chaos, Solitons & Fractals 155: 111694.
- [29] N. A. Kudryashov, (2022) “Exact solutions of equation for description of embedded solitons" Optik 268: 169801.
- [30] G. Wang, A.-M. Wazwaz, et al., (2022) “A new (3+ 1)- dimensional KdV equation and mKdV equation with their corresponding fractional forms" FRACTALS (fractals) 30(04): 1–8.
- [31] N. A. Kudryashov, (2022) “Stationary solitons of the generalized nonlinear Schrödinger equation with nonlinear dispersion and arbitrary refractive index" Applied Mathematics Letters 128: 107888.
- [32] Y. Fang, G.-Z. Wu, N. A. Kudryashov, Y.-Y. Wang, and C.-Q. Dai, (2022) “Data-driven soliton solutions and model parameters of nonlinear wave models via the conservation-law constrained neural network method" Chaos, Solitons & Fractals 158: 112118.
- [33] N. A. Kudryashov, (2022) “Bright and dark solitons in a nonlinear saturable medium" Physics Letters A 427: 127913.
- [34] W. B. Rabie and H. M. Ahmed, (2022) “Optical solitons for multiple-core couplers with polynomial law of nonlinearity using the modified extended direct algebraic method" Optik 258: 168848.
- [35] A. Houwe, M. Inc, S. Doka, B. Acay, and L. Hoan, (2020) “The discrete tanh method for solving the nonlinear differential-difference equations" International Journal of Modern Physics B 34(19): 2050177.
- [36] T. B. Benjamin, J. L. Bona, and J. J. Mahony, (1972) “Model equations for long waves in nonlinear dispersive systems" Philosophical Transactions of the Royal Society of London. Series A, Mathematical and Physical Sciences 272(1220): 47–78.
- [37] M. Osman, B. Ghanbari, and J. Machado, (2019) “New complex waves in nonlinear optics based on the complex Ginzburg-Landau equation with Kerr law nonlinearity" The European Physical Journal Plus 134(1): 1–10.
- [38] T. B. Benjamin, J. L. Bona, and J. J. Mahony, (1972) “Model equations for long waves in nonlinear dispersive systems" Philosophical Transactions of the Royal Society of London. Series A, Mathematical and Physical Sciences 272(1220): 47–78.
- [39] C. M. Khalique and K. R. Adem, (2011) “Exact solutions of the (2+ 1)-dimensional Zakharov–Kuznetsov modified equal width equation using Lie group analysis" Mathematical and Computer Modelling 54(1-2):184–189.
- [40] A.-M.Wazwaz, (2008) “Solitons and singular solitons for the Gardner–KP equation" Applied Mathematics and Computation 204(1): 162–169.
- [41] S. M. Mirhosseini-Alizamini, N. Ullah, J. Sabi’u, H. Rezazadeh, and M. Inc, (2021) “New exact solutions for nonlinear Atangana conformable Boussinesq-like equations by new Kudryashov method" International Journal of Modern Physics B 35(12): 2150163.