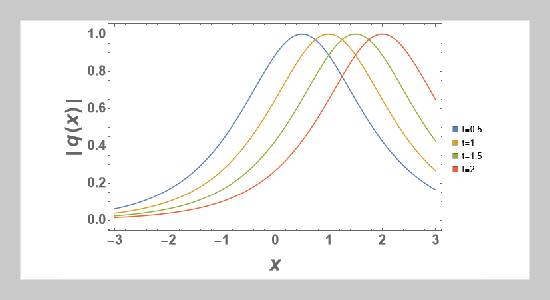
Yakup Yıldırım1,2, Anjan Biswas3,4,5,6This email address is being protected from spambots. You need JavaScript enabled to view it., Asim Asiri4
1Department of Computer Engineering, Biruni University, 34010 Istanbul, Turkey
2Department of Mathematics, Near East University, 99138 Nicosia, Cyprus
3Department of Mathematics and Physics, Grambling State University, Grambling, LA 71245-2715, USA
4Mathematical Modeling and Applied Computation (MMAC) Research Group, Center of Modern Mathematical Sciences and their Applications (CMMSA), Department of Mathematics, King Abdulaziz University, Jeddah–21589, Saudi Arabia
5Department of Applied Sciences, Cross–Border Faculty of Humanities, Economics and Engineering, Dunarea de Jos University of Galati, 111 Domneasca Street, Galati–800201, Romania
6Department of Mathematics and Applied Mathematics, Sefako Makgatho Health Sciences University, Medunsa–0204, South Africa
- [1] C. Chen, L. Li, and W. Liu, (2022) “Some New Optical Solitons of the Generalized Radhakrishnan–Kundu– Lakshmanan Equations with Powers of Nonlinearity" Symmetry 14(12): 2626. DOI: 10.3390/sym14122626.
- [2] N. A. Kudryashov, (2021) “The Radhakrishnan–Kundu– Lakshmanan equation with arbitrary refractive index and its exact solutions" Optik 238: 166738. DOI: 10.1016/j.ijleo.2021.166738.
- [3] N. A. Kudryashov, (2022) “Solitary waves of the generalized Radhakrishnan-Kundu-Lakshmanan equation with four powers of nonlinearity" Physics Letters A 448: 128327. DOI: 10.1016/j.physleta.2022.128327.
- [4] A. Neirameh, (2018) “Soliton solutions modeling of generalized Radhakrishnan-Kundu-Lakshmanan equation" Iranian Journal of Applied Physics 8(1): 71–80.
- [5] M. Kaplan and R. T. Alqahtani, (2023) “Exploration of New Solitons for the Fractional Perturbed Radhakrishnan– Kundu–Lakshmanan Model" Mathematics 11(11): 2562. DOI: 10.3390/math11112562.
- [6] N. Ozdemir, H. Esen, A. Secer, M. Bayram, T. A. Sulaiman, A. Yusuf, and H. Aydin, (2021) “Optical solitons and other solutions to the Radhakrishnan-KunduLakshmanan equation" Optik 242: 167363. DOI: 10.1016/j.ijleo.2021.167363.
- [7] A. H. Arnous, A. Biswas, Y. Yildirim, L. Moraru, M. Aphane, S. P. Moshokoa, and H. M. Alshehri, (2023) “Quiescent optical solitons with Kudryashov’s generalized quintuple-power and nonlocal nonlinearity having nonlinear chromatic dispersion: generalized temporal evolution" Ukr. J. Phys. Opt 24(2): 105.
- [8] A. H. Arnous, A. Biswas, Y. Yıldırım, L. Moraru, S. Moldovanu, and S. P. Moshokoa, (2022) “Quiescent Optical Solitons with Cubic–Quartic and Generalized Cubic–Quartic Nonlinearity" Electronics 11(22): 3653. DOI: 10.3390/electronics11223653.
- [9] A. H. Arnous, T. A. Nofal, A. Biswas, S. Khan, and L. Moraru, (2022) “Quiescent optical solitons with Kudryashov’s generalized quintuple-power and nonlocal nonlinearity having nonlinear chromatic dispersion" Universe 8(10): 501. DOI: 10.3390/universe8100501.
- [10] A. R. Adem, B. P. Ntsime, A. Biswas, M. Ekici, Y. Yildirim, and H. M. Alshehri, (2022) “Implicit quiescent optical solitons with complex Ginzburg–Landau equation having nonlinear chromatic dispersion" Journal of Optoelectronics and Advanced Materials 24(September-October 2022): 450–462.
- [11] Y. Yıldırım, A. Biswas, L. Moraru, and A. A. Alghamdi, (2023) “Quiescent optical solitons for the concatenation model with nonlinear chromatic dispersion" Mathematics 11(7): 1709. DOI: 10.3390/math11071709.
- [12] A. H. Arnous, A. Biswas, Y. Yıldırım, L. Moraru, S. Moldovanu, and A. A. Alghamdi, (2023) “Quiescent optical solitons with Kudryashov’s law of nonlinear refractive index" Results in Physics 47: 106394. DOI: 10.1016/j.rinp.2023.106394.
- [13] A. H. Arnous, A. Biswas, Y. Yıldırım, L. Moraru, S. Moldovanu, C. Iticescu, S. Khan, and H. M. Alshehri. “Quiescent Optical Solitons with Quadratic-Cubic and Generalized Quadratic-Cubic Nonlinearities”. In: Telecom. 4. 1. MDPI. 2023, 31–42. DOI: 10.3390/telecom4010003.
- [14] A. H. Arnous, A. Biswas, Y. Yıldırım, L. Moraru, S. Moldovanu, and A. A. Alghamdi, (2023) “Quiescent optical solitons with complex Ginzburg–Landau equation having a dozen forms of self–phase modulation" Heliyon 9(5): DOI: 10.1016/j.heliyon.2023.e15661.
- [15] M. Ekici, (2023) “Stationary optical solitons with Kudryashov’s quintuple power law nonlinearity by extended Jacobi’s elliptic function expansion" Journal of Nonlinear Optical Physics & Materials 32(01): 2350008.
- [16] M. Ekici, (2022) “Stationary optical solitons with complex Ginzburg–Landau equation having nonlinear chromatic dispersion and Kudryashov’s refractive index structures" Physics Letters A 440: 128146. DOI: 10.1016/j.physleta.2022.128146.
- [17] A. M. Yalçı and M. Ekici, (2022) “Stationary optical solitons with complex Ginzburg–Landau equation having nonlinear chromatic dispersion" Optical and Quantum Electronics 54(3): 167.
- [18] M. Mirzazadeh, M. Ekici, A. Sonmezoglu, M. Eslami, Q. Zhou, A. H. Kara, D. Milovic, F. B. Majid, A. Biswas, and M. Beli´c, (2016) “Optical solitons with complex Ginzburg–Landau equation" Nonlinear Dynamics 85: 1979–2016.
- [19] A. Yusuf, T. A. Sulaiman, E. Khalil, M. Bayram, and H. Ahmad, (2021) “Construction of multi-wave complexiton solutions of the Kadomtsev-Petviashvili equation via two efficient analyzing techniques" Results in Physics 21: 103775. DOI: 10.1016/j.rinp.2020.103775.
- [20] M. Cinar, A. Secer, M. Ozisik, and M. Bayram, (2022) “Derivation of optical solitons of dimensionless FokasLenells equation with perturbation term using Sardar sub-equation method" Optical and Quantum Electronics 54(7): 402. DOI: 10.1007/s11082-022-03819-0.
- [21] Z. Korpinar, M. Inc, D. Baleanu, and M. Bayram, (2019) “Theory and application for the time fractional Gardner equation with Mittag-Leffler kernel" Journal of Taibah University for Science 13(1): 813–819. DOI: 10.1080/16583655.2019.1640446.
- [22] B. Ibis, M. Bayram, and A. G. Agargun, (2011) “Applications of fractional differential transform method to fractional differential-algebraic equations" European Journal of Pure and Applied Mathematics 4(2): 129–141.
- [23] M. Ekici, (2022) “Kinky breathers, W-shaped and multipeak soliton interactions for Kudryashov’s quintuple power-law coupled with dual form of non-local refractive index structure" Chaos, Solitons & Fractals 159: 112172. DOI: 10.1016/j.chaos.2022.112172.
- [24] M. Ekici, (2022) “Optical solitons with Kudryashov’s quintuple power–law coupled with dual form of non–local law of refractive index with extended Jacobi’s elliptic function" Optical and Quantum Electronics 54(5): 279. DOI: 10.1007/s11082-022-03657-0.
- [25] M. Bayram, (2022) “Optical bullets with Biswas–Milovic equation having Kerr and parabolic laws of nonlinearity" Optik 270: 170046. DOI: 10.1016/j.ijleo.2022.170046.
- [26] T. Belyaeva, M. Agüero, and V. Serkin, (2021) “Nonautonomous solitons of the novel nonlinear Schrödinger equation: Self-compression, amplification, and the bound state decay in external potentials" Optik 244: 167584. DOI: 10.1016/j.ijleo.2021.167584.
- [27] N. A. Kudryashov, (2022) “Embedded Solitons of the Generalized Nonlinear Schrödinger Equation with High Dispersion" Regular and Chaotic Dynamics 27(6): 680–696. DOI: 10.1134/S1560354722060065.
- [28] A. Secer, (2022) “Stochastic optical solitons with multiplicative white noise via Itô calculus" Optik 268: 169831. DOI: 10.1016/j.ijleo.2022.169831.
- [29] N. Taghizadeh, M. Mirzazadeh, and A. S. Paghaleh, (2013) “Exact solutions of some nonlinear evolution equations via the first integral method" Ain Shams Engineering Journal 4(3): 493–499. DOI: 10.1016/j.asej.2012.10.002.
- [30] M.-Y. Wang, (2022) “Optical solitons with perturbed complex Ginzburg–Landau equation in Kerr and cubic– quintic–septic nonlinearity" Results in Physics 33: 105077. DOI: 10.1016/j.rinp.2021.105077.
- [31] A.-M. Wazwaz, (2021) “Bright and dark optical solitons for (3+ 1)-dimensional Schrödinger equation with cubic– quintic-septic nonlinearities" Optik 225: 165752.
- [32] A.-M. Wazwaz, (2018) “Two-mode Sharma-Tasso-Olver equation and two-mode fourth-order Burgers equation: Multiple kink solutions" Alexandria engineering journal 57(3): 1971–1976. DOI: 10.1016/j.aej.2017.04.003.
- [33] H. Wang, Q. Zhou, and W. Liu, (2022) “Exact analysis and elastic interaction of multi-soliton for a two-dimensional Gross-Pitaevskii equation in the BoseEinstein condensation" Journal of Advanced Research 38: 179–190. DOI: 10.1016/j.jare.2021.09.007.
- [34] T.-Y. Wang, Q. Zhou, and W.-J. Liu, (2022) “Soliton fusion and fission for the high-order coupled nonlinear Schrödinger system in fiber lasers" Chinese Physics B 31(2): 020501. DOI: 10.1088/1674-1056/ac2d22.
- [35] Y. Zhong, H. Triki, and Q. Zhou, (2023) “Analytical and numerical study of chirped optical solitons in a spatially inhomogeneous polynomial law fiber with paritytime symmetry potential" Communications in Theoretical Physics 75(2): 025003. DOI: 10.1088/1572-9494/aca51c.
- [36] Q. Zhou, D. Kumar, M. Mirzazadeh, M. Eslami, and H. Rezazadeh, (2018) “Optical soliton in nonlocal nonlinear medium with cubic-quintic nonlinearities and spatio-temporal dispersion" Acta Phys. Pol. A 134(6): 1204–1210. DOI: 10.12693/APhysPolA.134.1204.
- [37] Q. Zhou, H. Rezazadeh, A. Korkmaz, M. Eslami, M. Mirzazadeh, and M. Rezazadeh, (2019) “New optical solitary waves for unstable Schrödinger equation in nonlinear medium" Optica Applicata 49(1): 135–150. DOI: 10.5277/oa190112.
- [38] Q. Zhou, (2022) “Influence of parameters of optical fibers on optical soliton interactions" Chinese Physics Letters 39(1): 010501. DOI: 10.1088/0256-307X/39/1/010501.
- [39] Q. Zhou, Y. Sun, H. Triki, Y. Zhong, Z. Zeng, and M. Mirzazadeh, (2022) “Study on propagation properties of one-soliton in a multimode fiber with higher-order effects" Results in Physics 41: 105898. DOI: 10.1016/j.rinp.2022.105898.
- [40] Q. Zhou, Z. Luan, Z. Zeng, and Y. Zhong, (2022) “Effective amplification of optical solitons in high power transmission systems" Nonlinear Dynamics 109(4): 3083– 3089. DOI: 10.1007/s11071-022-07590-5.