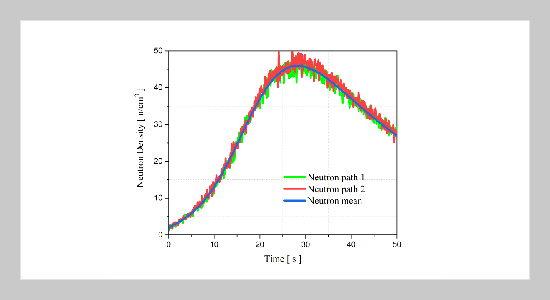
- [1] D.Hetrick. Dynamics of Nuclear Reactors. 1971.
- [2] N. Fujisawa, S. Liu, and T. Yamagata, (2021) “Nu merical study on ignition and failure mechanisms of hy drogen explosion accident in Fukushima Daiichi Unit 1" Engineering Failure Analysis 124: 105388. DOI: 10.1016/j.engfailanal.2021.105388.
- [3] Y. Lu, R. Zhu, X. Wang, C. An, Y. Zhao, and Q. Fu, (2019) “Experimental study on transient performance in the coasting transition process of shutdown for reactor coolant pump" Nuclear Engineering and Design 346: 192–199. DOI: 10.1016/j.nucengdes.2019.03.007.
- [4] P. P. Sanchez and A. dos Santos, (2021) “Prediction of the power peaking factor in a boron-free small modular reactor based on a support vector regression model and control rod bank positions" Nuclear Science and Engi neering 195(5): 555–562. DOI: 10.1080/00295639.2020.1854541.
- [5] W. M. Stacey. Nuclear reactor physics. John Wiley & Sons, 2018.
- [6] T. Gordon, M. Williams, M. Eaton, and P. Haigh, (2024) “Itô-calculus based mathematical models for stochastic nuclear reactor kinetics and dynamics simu lations of low neutron source nuclear power plant (NPP) start-up" Annals of Nuclear Energy 202: 110451. DOI: 10.1016/j.anucene.2024.110451.
- [7] J. G. Hayes and E. Allen, (2005) “Stochastic point kinetics equations in nuclear reactor dynamics" Annals of nuclear energy 32(6): 572–587. DOI: 10.1016/j.anucene.2004.11.009.
- [8] S. S. Ray, (2012) “Numerical simulation of stochastic point kinetic equation in the dynamical system of nuclear reactor" Annals of Nuclear Energy 49: 154–159. DOI: 10.1016/j.anucene.2012.05.022.
- [9] S. Saha Ray and A. Patra, (2013) “Numerical solution for stochastic point-kinetics equations with sinusoidal re activity in dynamical system of nuclear reactor" Inter national Journal of Nuclear Energy Science and Technology 7(3): 231–242. DOI: 10.1504/IJNEST.2013.052165.
- [10] S. M. Ayyoubzadeh and N. Vosoughi, (2014) “An alternative stochastic formulation for the point reactor" Annals of Nuclear Energy 63: 691–695. DOI: 10.1016/j.anucene.2013.09.013.
- [11] A.NahlaandA.Edress,(2016) “Analytical exponential model for stochastic point kinetics equations via eigen values and eigenvectors" Nuclear Science and Tech niques 27: 1–8. DOI: 10.1007/s41365-016-0025-6.
- [12] A.A.NahlaandA.M.Edress,(2016)“Efficientstochas tic model for the point kinetics equations" Stochastic Analysis and Applications 34(4): 598–609. DOI: 10.1080/07362994.2016.1159519.
- [13] M.W.daSilva, R. Vasques, B. E. Bodmann, and M. T. Vilhena, (2016) “A nonstiff solution for the stochastic neutron point kinetics equations" Annals of Nuclear Energy 97: 47–52. DOI: 10.1016/j.anucene.2016.06.026.
- [14] D. Suescún-Díaz, Y. M. Oviedo-Torres, and L. E. Girón-Cruz, (2018) “Solution of the stochastic point kinetics equations using the implicit Euler-Maruyama method" Annals of Nuclear Energy 117: 45–52. DOI: 10.1016/j.anucene.2018.03.013.
- [15] M. El-Beltagy and A. Noor, (2019) “Analysis of the stochastic point reactor using Wiener-Hermite expansion" Annals of Nuclear Energy 134: 250–257. DOI: 10. 1016/j.anucene.2019.06.022.
- [16] S. Alaskary and M. El-Beltagy, (2020) “Uncertainty quantification spectral technique for the stochastic point reactor with random parameters" Energies 13(6): 1297. DOI: 10.3390/en13061297.
- [17] A. Elsayed, M. El-Beltagy, A. Al-Juhani, and S. Al Qahtani, (2021) “A new model for the stochastic point reactor: Development and comparison with available mod els" Energies 14(4): 955. DOI: 10.3390/en14040955.
- [18] A. Elsayed and M. El-Beltagy, (2022) “An efficient space-time model for the stochastic nuclear reactors" An nals of Nuclear Energy 169: 108921. DOI: 10.1016/j.anucene.2021.108921.
- [19] A.AboanberandY.Hamada,(2002)“PWS:anefficient code system for solving space-independent nuclear reactor dynamics" Annals of Nuclear Energy 29(18): 2159 2172. DOI: 10.1016/S0306-4549(02)00034-8.
- [20] A. Aboanber and Y. Hamada, (2003) “Power series solution (PWS) of nuclear reactor dynamics with New tonian temperature feedback" Annals of Nuclear En ergy 30(10): 1111–1122. DOI: 10.1016/S0306-4549(03)00033-1.
- [21] B. Ganapol, P. Picca, A. Previti, D. Mostacci, et al., (2012) “The solution of the point kinetics equations via converged accelerated Taylor series (CATS)" Proceed ings of PHYSOR2012:
- [22] P. Picca, R. Furfaro, and B. D. Ganapol, (2013) “A highly accurate technique for the solution of the non-linear point kinetics equations" Annals of Nuclear Energy 58: 43–53. DOI: 10.1016/j.anucene.2013.03.004.
- [23] A. Patra and S. S. Ray, (2015) “On the solution of the nonlinear fractional neutron point-kinetics equation with Newtonian temperature feedback reactivity" Nu clear Technology 189(1): 103–109. DOI: 10.13182/NT13-148.
- [24] S. Q. B. Leite, M. T. de Vilhena, and B. E. Bodmann, (2016) “Solution of the point reactor kinetics equations with temperature feedback by the ITS2 method" Progress in Nuclear Energy 91: 240–249. DOI: 10.1016/j.pnucene.2016.05.001.
- [25] D. Suescún-Díaz, D. A. Rasero-Causil, and J. H. Lozano-Parada, (2019) “Neutron density calculation using the generalised adams-bashforth-moulton method" Universitas Scientiarum 24(3): 543–563. DOI: 10.11144/Javeriana.SC24-3.ndcu.
- [26] D. Suescún-Díaz and G. Espinosa-Paredes, (2020) “On the numerical solution of the point reactor kinet ics equations" Nuclear Engineering and Technology 52(6): 1340–1346. DOI: 10.1016/j.net.2019.11.034.
- [27] A. A. Nahla, (2011) “An efficient technique for the point reactor kinetics equations with Newtonian temperature feedback effects" Annals of Nuclear Energy 38(12): 2810–2817. DOI: 10.1016/j.anucene.2011.08.021.
- [28] S.SinghandS.S.Ray,(2017)“Onthecomparisonoftwo split-step methods for the numerical simulation of stochas tic point kinetics equations in presence of Newtonian tem perature feedback effects" Annals of Nuclear Energy 110: 865–873. DOI: 10.1016/j.anucene.2017.08.001.
- [29] S. S. Ray and S. Singh, (2019) “Numerical solutions of stochastic nonlinear point reactor kinetics equations in presence of Newtonian temperature feedback effects" Jour nal of Computational and Theoretical Transport 48(2): 47–57. DOI: 10.1080/23324309.2019.1604549.
- [30] J. Hyvärinen, J. Vihavainen, M. Ylönen, and J. Valko nen, (2022) “An overall safety concept for nuclear power plants" Annals of Nuclear Energy 178: 109353. DOI: 10.1016/j.anucene.2022.109353.
- [31] N.Hossain, M.A.M.Soner, M.M.H.Prodhan,M.H. Sahadath, and K. A. Kabir, (2022) “Experimental anal ysis of step reactivity insertion effect on reactor power, fuel temperature and reactor period in BAEC TRIGA research reactor" Annals of Nuclear Energy 165: 108665. DOI: 10.1016/j.anucene.2021.108665.
- [32] W.Jiang, Q.-F. Zhu, Q. Zhou, F. Ma, L. Zhang, Y. Liu, J.-Y. Li, H.-L. Ge, R. Yu, H.-Y. Meng, et al., (2022) “Re activity worth measurement of the lead target on VENUS II light water reactor and validation of evaluated nuclear data" Annals of Nuclear Energy 165: 108779. DOI: 10.1016/j.anucene.2021.108779.
- [33] V. Vasilenko, A. Pankin, and K. Skvortsov, (2019) “Reactivity Calibrator" Atomic Energy 125(3): 157–161. DOI: 10.1007/s10512-018-00459-0.
- [34] V. Zhitarev, V. Kachanov, A. Y. Sergevnin, and N. Sutemieva, (2021) “Correction of the Reactivity Mea sured by ORUK Technique" Physics of Atomic Nuclei 84: 1405–1412. DOI: 10.1134/S1063778821080214.
- [35] D. Suescún-Díaz, G. Ule-Duque, and J. A. Chala Casanova, (2023) “Nuclear Reactivity Calculation with Reduction of Fluctuations." International Journal of Technology 14(5): DOI: 10.14716/ijtech.v14i5.5970.
- [36] G. B. Arfken, H. J. Weber, and F. E. Harris. Mathe matical methods for physicists: a comprehensive guide. Academic press, 2011.
- [37] P. E. Kloeden, E. Platen, P. E. Kloeden, and E. Platen. Stochastic differential equations. Springer, 1992.